Adam Crowl has been appearing on Centauri Dreams for almost as long as the site has been in existence, a welcome addition given his polymathic interests and ability to cut to the heart of any issue. His long-term interest in interstellar propulsion has recently been piqued by the Jet Propulsion Laboratory’s work on a mission to the Sun’s gravitational lens region. JPL is homing in on multiple sailcraft with close solar passes to expedite the cruise time, leading Adam to run through the options to illustrate the issues involved in so dramatic a mission. Today he looks at the pros and cons of nuclear propulsion, asking whether it could be used to shorten the trip dramatically. Beamed sail and laser-powered ion drive possibilities are slated for future posts. With each of these, if we want to get out past 550 AU as quickly as possible, the devil is in the details. To keep up with Adam’s work, keep an eye on Crowlspace.
by Adam Crowl

The Solar Gravitational Lens amplifies signals from distant stars and galaxies immensely, thanks to the slight distortion of space-time caused by the Sun’s mass-energy. Basically the Sun becomes an immense spherical lens, amplifying incoming light by focussing it hundreds of Astronomical Units (AU) away. Depending on the light frequency, the Sun’s surrounding plasma in its Corona can cause interference, so the minimum distance varies. For optical frequencies it can be ~600 AU at a minimum and light is usefully focussed out to ~1,000 AU.
One AU is traveled in 1 Julian Year (365.25 days) at a speed of 4.74 km/s. Thus to travel 100 AU in 1 year needs a speed of 474 km/s, which is much faster than the 16.65 km/s that probes have been launched away from the Earth. If a Solar Sail propulsion system could be deployed close to the Sun and have a Lifting Factor (the ratio of Light-Pressure to Weight of Solar Sail vehicle) greater than 1, then such a mission could be launched easily. However, at present, we don’t have super-reflective gossamer light materials that could usefully lift a payload against solar gravity.
Carbon nanotube mesh has been studied in such a context, as has aerographite, but both are yet to be created in large enough areas to carry large payloads. The ratio of the push of sunlight, for a perfect reflector, to the gravity of the Sun means an areal mass density of 1.53 grams per square metre gives a Lifting Factor of 1. A Sail with such an LF will hover when pointing face on at the Sun. If a Solar Sail LF is less than 1, then it can be angled and used to speed up or slow down the Sail relative to its initial orbital vector, but the available trajectories are then slow spirals – not fast enough to reach the Gravity Lens in a useful time.
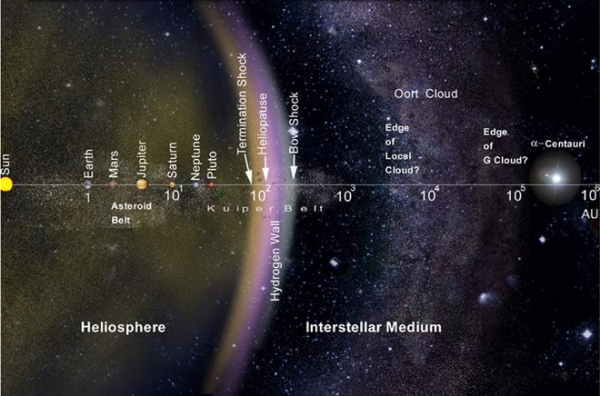
Image: A logarithmic look at where we’d like to go. Credit: NASA.
Absent super-light Solar Sails, what are the options? Modern day rockets can’t reach 474 km/s without some radical improvements. Multi-grid Ion Drives can achieve exhaust velocities of the right scale, but no power source yet available can supply the energy required. The reason why leads into the next couple of options so it’s worth exploring. For deep space missions the only working option for high-power is a nuclear fission reactor, since we’re yet to build a working nuclear fusion reactor.
When a rocket’s thrust is limited by the power supply’s mass, then there’s a minimum power & minimum travel time trajectory with a specific acceleration/deceleration profile – it accelerates 1/3 the time, then cruises at constant speed 1/3 the time, then brakes 1/3 the time. The minimum Specific Power (Power per kilogram) is:
P/M = (27/4)*S2*T-3
…where P/M is Power/Mass, S is displacement (distance traveled) and T is the total mission time to travel the displacement S. In units of AU and Years, the P/M becomes:
P/M = 4.8*S2*T-3 W/kg
However while the Average Speed is 474 km/s for a 6 year mission to 600 AU, the acceleration/deceleration must be accounted for. The Cruise Speed is thus 3/2 times higher, so the total Delta-Vee is 3 times the Average Speed. The optimal mass-ratio for the rocket is about 4.41, so the required Effective Exhaust Velocity is a bit over twice the Average Speed – in this case 958 km/s. As a result the energy efficiency is 0.323, meaning the required Specific Power for a rocket is:
P/M = 14.9*S2*T-3 W/kg
For a mission to 600 AU in 6 years a Specific Power of 24,850 W/kg is needed. But this is the ideal Jet-Power – the kinetic energy that actually goes into the forward thrust of the vehicle. Assuming the power source is 40% (40% drive and 10% payload) of the vehicle’s empty mass and the efficiency of the higher-powered multi-grid ion-drive is 80%, then the power source must produce 77,600 W/kg of power. Every power source produces waste heat. For a fission power supply, the waste heat can only be expelled by a radiator. Thermodynamic efficiency is defined as the difference in temperature between the heat-source (reactor) and the heat-sink (radiator), divided by the temperature of the heat source:
Thermal Efficiency = (Tsource – Tsink) / Tsource
For a reactor with a radiator in space, the mass of that radiator is (usually) minimised when the efficiency is 25 % – so to maximise the Power/Mass ratio the reactor has to be really HOT. The heat of the reactor is carried away into a heat exchanger and then travels through the radiator to dump the waste heat to space. To minimise mass and moving parts so called Heat-Pipes can be used, which are conductive channels of certain alloys.
Another option, which may prove highly effective given clever reactor designs, is to use high performance thermophotovoltaic (TPV) cells to convert high temperature thermal emissions directly into electrical power. High performance TPV’s have hit 40% efficiency at over 2,000 degrees C, which would also maximise the P/M ratio of the whole power system.
Pure Uranium-235, if perfectly fissioned (a Burn-Up Fraction of 1), releases 88 trillion joules (88 TJ) per kilogram. A jet-power of 24,850 W/kg sustained for 4 years is a total power output of 3.1 TJ/kg. Operating the Solar Lens Telescope payload won’t require such power levels, so we’ll assume it’s negligible fraction of the total output – a much lower power setting. So our fuel needs to be *at least* 3.6% Uranium-235. But there’s multipliers which increase the fraction required – not all the vehicle will be U-235.
First, the power-supply mass fraction and the ion-drive efficiency – a multiplier of 1/0.32. Therefore the fuel must be 11.1% U-235.
Second, there’s the thermodynamic efficiency. To minimise the radiator area (thus mass) required, it’s set at 25%. Therefore the U-235 is 45.6% of the power system mass. The Specific Power needed for the whole system is thus 310,625 W per kilogram.
The final limitation I haven’t mentioned until now – the thermophysical properties of Uranium itself. Typically Uranium is in the form of Uranium Dioxide, which is 88% uranium by mass. When heated every material goes up in temperature by absorbing (or producing internally) a certain amount of heat – the so called Heat Capacity. The total amount of heat stored in a given amount of material is called the Enthalpy, but what matters to extracting heat from a mass of fissioning Uranium is the difference in Enthalpy between a Higher and a Lower temperature.
Considering the whole of the reactor core and the radiator as a single unit, the Lower temperature will be the radiator temperature. The Higher will be the Core where it physically contacts the heat exchanger/radiator. Thanks to the Thermal efficiency relation we know that if the radiator is at 2,000 K, then the Core must be at least ~2,670 K. The Enthalpy difference is 339 kilojoules per kilogram of Uranium Oxide core. Extracting that heat difference every second maintains the temperature difference between the Source and the Sink to make Work (useful power) and that means a bare minimum of 91.6% of the specific mass of the whole power system must be very hot fissioning Uranium Dioxide core. Even if the Core is at melting point – about 3120 K – then the Enthalpy difference is 348 KJ/kg – 89.3% of the Power System is Core.
The trend is obvious. The power supply ends up being almost all fissioning Uranium, which is obviously absurd.
To conclude: A fission powered mission to 600 AU will take longer than 6 years. As the Power required is proportional to the inverse cube of the mission time, the total energy required is proportional to the inverse square of the mission time. So a mission time of 12 years means the fraction of U-235 burn-up comes down to a more achievable 22.9% of the power supply’s total mass. A reactor core is more than just fissioning metal oxide. Small reactors have been designed with fuel fractions of 10%, but this is without radiators. A 5% core mass puts the system in range of a 24 year mission time, but that’s approaching near term Solar Sail performance.



I believe Dr Winterburg had a look in the 70’s for micro fission explosions which we could be use for propulsion. After laser compression of the fuel pellet another laser could be used to generate neutrons which are directed towards the compressed pellet say via movable nanotubes to guide the neutrons to the highly compressed pellet, timing is essential. A magnetic field is then used to direct the explosion out of the nozzle providing thrust.
https://www.degruyter.com/document/doi/10.1515/zna-1973-0610/html?lang=en
Hi Michael
Electron beam compression – and laser – has not been demonstrated for *small* systems. While there’s advances in electron beams all the time, the power required for such compression requires some heavy auxillary systems – like massive capacitor banks.
Another option looked at since the early 2000’s is micro-fission explosions set-off via Z-pinch – this has been studied by Dana Andrews and colleagues under the name of Mini-Mag Orion. Any externally detonated system is not as dramatically affected by heat as power-source driven systems like ion-drives. The bulk of the heat is dumped straight to space and, of course, the enthalpy of a fissioning plasma is much, much higher than a solid-core reactor.
Which is a natural segue into that perennial obsession of fission enthusiasts, the Plasma Core Reactor. Sadly what is essentially a barely contained continuous nuclear explosion makes a lot of people very, very nervous and probably won’t see experimental research until we’re well established in space.
Theses calculations and experiments would have been carried out already to test nuclear weapon effectiveness. Even if the fissile material does not undergo complete fission even a small percentage would exceed anything we have today. As for the power needed potentially we could use a phased laser array like starshot to power the craft during acceleration.
Hi Michael
I’m a fan of space nuclear power, but anything requiring fission of sub-critical masses requires big heavy machines. Not a space-probe scale mass. And unlikely to achieve a high Power-to-Mass ratio, unless the vehicle itself is very large (+100 tons).
Though, as one of my replies below discusses, that’s not such a constraint if we have a supply of antimatter. Paul Gilster has featured various antimatter schemes here before, so I won’t elaborate further.
As for laser phased arrays, Paul’s intro indicated that’s the topic of a future post I’m working on. NASA has done a lot of work on the concept. But what are all the options?
Adam
The key take away is that we don’t need enormous compression of the fission material because we can use the neutron generator/nanotube focus powered by the laser to produce many neutrons to induce fission. The phased array gives the probe more than enough energy to drive the generator.
“Theses calculations and experiments would have been carried out already to test nuclear weapon effectiveness. Even if the fissile material does not undergo complete fission even a small percentage would exceed anything we have today.”
no matter what the percentage is, if its outside the ship the waste heat problem disappears
Carlo Rubbia seemed to think Americium was the answer. Now I wonder if a cross between autophage rockets, NSWR, and Orion shaped charges might allow a low tech, almost SRB type burn that replaces electronics for plumbing, as it were.
ission
The isotope 242mAm (half-life 141 years) has the largest cross sections for absorption of thermal neutrons (5,700 barns),[90] that results in a small critical mass for a sustained nuclear chain reaction. The critical mass for a bare 242mAm sphere is about 9–14 kg (the uncertainty results from insufficient knowledge of its material properties). It can be lowered to 3–5 kg with a metal reflector and should become even smaller with a water reflector.[91] Such small critical mass is favorable for portable nuclear weapons, but those based on 242mAm are not known yet, probably because of its scarcity and high price. The critical masses of two other readily available isotopes, 241Am and 243Am, are relatively high – 57.6 to 75.6 kg for 241Am and 209 kg for 243Am.[92] Scarcity and high price yet hinder application of americium as a nuclear fuel in nuclear reactors.[93]
There are proposals of very compact 10-kW high-flux reactors using as little as 20 grams of 242mAm. Such low-power reactors would be relatively safe to use as neutron sources for radiation therapy in hospitals.[94]
Hi Adam, are you going to cover the Windrider is this series? By its very nature it cannot do the six years objective, but eight years is not so bad… https://agu.confex.com/agu/fm21/meetingapp.cgi/Paper/796237
If you want to have a long range project with support use the same technology to stop asteroids from impacting on earth. Any high power system could also be used for planetary defense and that is where you will receive funding, protecting the earth first. Plus politicians just love that… ;-}
Apart from the raw propulsion required, the mission objectives come into play. Let’s assume a fly-by mission.
Being very approximate, the duration of the mission is ~50% of the direct travel time. The mission ends when the observatory is too far out. If it takes 20 years to reach 550-600 AU, the mission lasts about 10 years.
During the mission, propulsion is only needed for modest lateral motion to accurately aim at the target. Small satellite observatories may be needed for effective data acquisition. Those can be deployed when 550 AU is achieved.
Don’t expect to have more than a few observation targets since, at that distance from the Sun, there is only a small portion of the sky that is accessible. 10 years may be more than enough for what can be reasonably achieved. Indeed, a fraction of that may be all that’s needed.
The propulsion implication is that a deceleration phase is likely unnecessary. Travel outbound as fast as possible with conceivable propulsion technologies and there is almost certainly enough time before passing 1000 AU for all the science objectives to be met.
That narrows the requirements for spacecraft propulsion. It will not be easier, just a more focused effort. Getting a functioning, long-lived observatory to the gravitational focus remains a severe challenge.
Hi Ron
Sadly a gravity lens will have a very narrow field of view indeed. Likely a single planet or compact star system with planets in the same plane. Maybe the Galactic Core? But as the useful lensing distance is a radial range about 450 AU long, then some speed is ok, or even useful for tracking the target as it orbits its star.
Interstellar probe: Has its time finally come?
By Leonard David published about 7 hours ago
“It isn’t about where we are going. It’s about the journey out there. And it is a journey now long overdue.”
https://www.space.com/interstellar-probe-johns-hopkins-apl-nasa-sls?utm_campaign=58E4DE65-C57F-4CD3-9A5A-609994E2C5A9
Speaking of the nuclear option…
https://centauri-dreams.org/2016/09/16/project-orion-a-nuclear-bomb-and-rocket-all-in-one/
Some interesting heuristics for a nuclear-powered ion engine that I haven’t seen before.
Although you seem unhappy that a slower velocity and longer mission time is not really any improvement over the 25 AU/yr sail with a sundiver trajectory, what would the reactor need to be to supply the energy for a similar velocity with no deceleration? The advantage of the ion-drive is that the craft would not need to do the risky sundiver maneuver, and the multiple telescopes could be deployed as the craft reaches its destination. This strikes me as a less risky mission if the necessary power can be supplied and the mass ratio of the system looks reasonable.
I look forward to your analysis of using beamed power instead of a reactor, although how you hope the beams will be sufficient to decelerate the craft will be interesting.
I also wonder whether a hybrid energy supply offers a better approach, e.g. beams for the acceleration period, and a reactor for the deceleration.
Adam and Paul – thank you for a lovely concise take on the subject. I am reminded when reading such theses of the 1989 New Scientist article on antimatter propulsion by the inimitable Joel Davis (with Antimatter To The Stars). I cannot provide a link but am happy to send a scanned copy to anyone interested.
Hi David
Antimatter has another use which that 1989 article didn’t consider – it’s almost perfect for causing nuclear fission. Small amounts can be used to fission much larger fuel pellets in an external propulsion system. No Z-Pinch or e-beams or lasers required. Of course it’s there’s a fissioning plasma to be directed and lots of x-rays and neutrons. Very unhealthy near other vehicles. But in theory very effective.
That Joel Davis article online here, complete with some cool space artwork:
https://books.google.com/books?id=t6k3RHx52_cC&pg=PA66&lpg=PA66&dq=joel+davis+antimatter+to+the+stars+1989&source=bl&ots=kuAO6gFkgM&sig=ACfU3U1r6EH6H-qkYUzhdNReAR6pdkSDRA&hl=en&sa=X&ved=2ahUKEwi-2JPUo5n5AhWzFlkFHVM6CZEQ6AF6BAgZEAM#v=onepage&q=joel%20davis%20antimatter%20to%20the%20stars%201989&f=false
And in part here:
https://www.newscientist.com/article/mg12216705-700-with-antimatter-to-the-stars/
Davis is also the co-author with Robert Forward in the book Mirror Matter: Pioneering Antimatter Physics:
https://www.isbns.net/isbn/9780595198177/
https://www.ans.org/pubs/journals/download/article-29376/
One big reason antimatter is currently so insanely expensive is that those institutions which do generate it are not doing it with any specific goal in mind in terms of what to do with that antimatter. If they were making it for propulsion, the prices could start going down and we might actually see it being used to get us to the stars. So much potential just sitting there because so many won’t or can’t look past their noses.
Adam, ljk, thank you for your responses. I wonder whether subsequent research provides a basis for an update to ‘Mirror Matter: Pioneering Antimatter Physics’? I have long thought that were we to definitively discover ‘possible only with life’ signatures in the atmosphere of a nearby exoplanet, interest in the most energetic propulsion systems would ‘explode’. Perhaps the Webb telescope will one day provide that evidence. Perhaps Paul, fortunate travellers in the not-too-distant future will bath in the light of the Alpha Centauri triple star system.
A wonderful thought, David, and I like the ‘not too distant future’ idea. Re antimatter, Gerald Jackson continues to push intriguing ideas on interstellar applications. Your comment makes me realize it’s time for me to catch up with Gerry and update us on his work.
David Herne, it may be possible to detect starships moving at FTL speeds thusly…
https://adsabs.harvard.edu/full/2005ICRC….5..387H
Title: Optical SETI with Imaging Cherenkov Telescopes
Authors: Holder, J., Ashworth, P., LeBohec, S., Rose, H. J., & Weekes, T. C.
Journal: Proceedings of the 29th International Cosmic Ray Conference. August 3-10, 2005, Pune, India. Edited by B. Sripathi Acharya, Sunil Gupta, P. Jagadeesan, Atul Jain, S. Karthikeyan, Samuel Morris, and Suresh Tonwar. Mumbai: Tata Institute of Fundamental Research, 2005. Volume 5, p.387
Bibliographic Code: 2005ICRC….5..387H
The paper is also online here:
https://arxiv.org/pdf/astro-ph/0506758.pdf
See also:
https://centauri-dreams.org/2012/08/21/seti-starship-radiation-signatures/
The connecting paper is here:
https://articles.adsabs.harvard.edu/full/1995ASPC…74..487Z
Title: Detection of Extraterrestrial Civilizations via the Spectral Signature of Advanced Interstellar Spacecraft
Authors: Zubrin, R.
Journal: Astronomical Society of the Pacific Conference Series, Volume 74. Progress in the Search for Extraterrestrial Life. 1993 Bioastronomy Symposium, held in Santa Cruz, California, August 16-20,1993. Editor, G. Seth Shostak; Publisher, Astronomical Society of the Pacific, San Francisco, California, 1995. LC # QB54 .P76 1993. ISBN # 0-937707-93-7., p.487
Bibliographic Code: 1995ASPC…74..487Z
Did I miss something? A quick perusal shows only discussion of sub-light speeds. In any case, it isn’t clear how one would do FTL detection since the physics is unknown (or impossible).
How far away can the target star be? If you can get, say 1 km resolution per parsec, would you be able to resolve gas giant disks in the Magellanic Clouds?
Perhaps a nuclear decay sail, the sail deploys and then a container of radioactive material is vapourised and sprayed onto one side of the sail where it sticks and undergoes radioctave decay for propulsion and energy generation.
To my imagination, the future applications of nuclear power are dominated by induced gamma emission or similar processes. That’s a long-simmering field that seems to offer only tantalizing hints and not much substance … still, is there a way? Imagine you could effectively route power into and out of nuclei, so that you could cause an isotope to release its power on demand. Then imagine you could mix that isotope with another that would absorb its high-energy gamma rays, then release them in smaller quanta while transitioning from one nuclear isomer to the next. Imagine you could set up a series of such isotopes in some spectacularly controlled environment, until the radioactive energy of a nucleus could be milked, at the rate you request, from a nuclear fuel source, and broken up into a succession of lower-frequency photons until it can be harvested directly by a semiconductor for electrical energy. A nuclear power source without dangerous radioactivity, even without much heat … is there any physics that proves it is not possible?
Adam, have you considered using nuclear/ion for acceleration only, then braking via magnetic or electro-static means?
Hi Dave
That would be telling. I discuss mag-sails and the like in future installments.
You mention : ‘it accelerates 1/3 the time, then cruises at constant speed 1/3 the time, then brakes 1/3 the time.’
I was under the impression the gravitational lensing telescope missions that need to reach +500 AU would not be slowly down as the further they go, the better the lensing affect, avoiding the sun’s corona etc.
If you don’t have to slow down, this should make the calculations look a little better, don’t you think?
Hi Chris
Some outward motion is perhaps acceptable, but 100 AU/year seems too high. Also not stopping a 100 AU/year cruise speed perhaps makes lateral maneuvers more difficult. However if that’s the option, then the total acceleration time is 2/3 the total travel time and the specific power is 1/4 the rendezvous mission.
Not stopping has no influence on lateral maneuvers at all. How exactly would it?
Hi Paul
A good summary reference for high performance Ion Drives is an IEPC paper by Bond & Martin (x2):
ULTIMATE PERFORMANCE LIMITS AND MISSION CAPABILITIES OF ADVANCED ION THRUSTERS
The JPL Starprobe design by Aston has a cruise speed of 0.0122 c and hits 1000 AU after 15 years of flight. Total acceleration time is 65 years. i.e. less than 2 milligees acceleration.
Adam, do you have the reference handy on Aston?
Hi Paul
The AIAA published the IEPC for 1985. Aston’s paper is here:
Electric propulsion – A far reaching technology
G. ASTON
https://doi.org/10.2514/6.1985-2028
It was also published in a 1986 JBIS.
“…and hits 1000 AU after 15 years of flight. Total acceleration time is 65 years.”
u saying that there is 15 yrs. of non-powered flight ? 80 yr. mission ?
Hi Charlie
No. It keeps accelerating. The Starprobe is a Proxima mission, but one of the points along the way is the 1,000 AU mark. I mentioned that by way of comparison. As it’s not a rendezvous mission, it keeps accelerating until burn-out at year 65, then coasts for the remainder of the 389 year flight to Proxima. The initial mass-ratio is 2.5 and the acceleration isn’t constant, but varies with the mass of propellant remaining. Average is 0.000182 gee. Less than 0.2 milligee (not 2 milligee, as I said above). The exhaust velocity is so high.
Adam
The Voyagers are old, not dead. And they are the only functioning deep space probes we have out that far. New Horizons is still well behind them and Pioneer 10 and 11 stopped transmitting a long time ago.
https://www.space.com/voyager-spacecraft-power-update-july-2022
Scientists’ predictions for the long-term future of the Voyager Golden Records will blow your mind
By Meghan Bartels
published February 23, 2021
Buckle up, everyone, and let’s take a ride on a universe-size time machine.
https://www.space.com/predicting-voyager-golden-records-distant-future
To quote:
By 500 million years from now, the solar system and the Voyagers alike will complete a full orbit through the Milky Way. There’s no way to predict what will have happened on Earth’s surface by then, but it’s a timespan on the scale of the formation and destruction of Pangaea and other supercontinents, Oberg said.
Throughout this galactic orbit, the Voyager spacecraft will oscillate up and down, with Voyager 1 doing so more dramatically than its twin. According to these models, Voyager 1 will travel so far above the main disk of the galaxy that it will see stars at just half the density as we do.
…
Both Golden Records have good odds of remaining legible, since their engraved sides are tucked away against the spacecraft bodies. The outer surface of Voyager 1’s record is more likely to erode away, but the information on Voyager 2’s record is more likely to become illegible, Oberg said.
“The main reason for this is because the orbit that Voyager 2 is flung into is more chaotic, and it’s significantly more difficult to predict with any certainty of exactly what sort of environment it’s going to be flying through,” he said.
But despite the onslaught and potential detours, “Both Golden Records are highly likely to survive at least partially intact for a span of over 5 billion years,” Oberg said.
…
Meanwhile, the vicarious sightseeing continues. Oberg and his colleague calculated that in this 5-billion-year model-friendly period, each of the Voyagers likely visits a star besides our sun within about 150 times the distance between Earth and the sun, or three times the distance between the sun and Pluto at the dwarf planet’s most distant point.
Precisely which star that might be, however, is tricky — it may not even be a star we know today.
“While neither Voyager is likely to get particularly close to any star before the galaxies collide, the craft are likely to at least pass through the outskirts of some [star] system,” Oberg said. “The very strange part is that that actually might be a system that does not yet exist, of a star that has yet to be born.”
Such are the perils of working on a scale of billions of years.
From here, the Voyagers’ fate depends on the conditions of the galactic merger, Oberg said.
The collision itself might kick a spacecraft out of the newly monstrous galaxy — a one in five chance, he said — although it would remain stuck in the neighborhood. If that occurs, the biggest threat to the Golden Records would become collisions with high-energy cosmic rays and the odd molecule of hot gas, Oberg said; these impacts would be rarer than the dust that characterized their damage inside the Milky Way.
Inside the combined galaxy, the Voyagers’ fate would depend on how much dust is left behind by the merger; Oberg said that may well be minimal as star formation and explosion both slow, reducing the amount of dust flung into the galaxy.
Depending on their luck with this dust, the Voyagers may be able to ride out trillions of trillions of trillions of years, long enough to cruise through a truly alien cosmos, Oberg said.
“Such a distant time is far beyond the point where stars have exhausted their fuel and star formation has ceased in its entirety in the universe,” he said. “The Voyagers will be drifting through what would be, to us, a completely unrecognizable galaxy, free of so-called main-sequence stars, populated almost exclusively by black holes and stellar remnants such as a white dwarfs and neutron stars.”
It’s a dark future, Oberg added. “The only source of significant illumination in this epoch will be supernovas that results from the once-in-a-trillion-year collision between these stellar remnants that still populate the galaxy,” he said. “Our work, found on these records, thus may bear witness to these isolated flashes in the dark.”
What Happens When NASA’s Voyager I And II Run Out Of Fuel?
NASA’s Voyager probes will continue their legacy in space despite running out of power and fuel, and are NASA’s longest-lasting mission.
BY GISELLE DUSSEL
PUBLISHED August 1, 2022
https://screenrant.com/what-happens-nasa-voyager-probes-no-fuel/
THE UNTOLD TRUTH OF THE VOYAGER PROGRAM
BY JOSEPH A. WILLIAMS/AUG. 2, 2022 2:44 PM EDT
https://www.grunge.com/949833/the-untold-truth-of-the-voyager-program