“‘This,’ said I at length, to the old man — ‘this can be nothing else than the great whirlpool of the Maelström’… The ordinary accounts of this vortex had by no means prepared me for what I saw. That of Jonas Ramus, which is perhaps the most circumstantial of any, cannot impart the faintest conception either of the magnificence, or of the horror of the scene — or of the wild bewildering sense of the novel which confounds the beholder.” So wrote Edgar Allen Poe in 1841 in a short story called “A Descent into The Maelström,” reckoned by some to be an early instance of science fiction. In today’s essay, Adam Crowl explores another kind of whirlpool, armed with the tools of mathematics to take the deepest plunge imaginable, into the maw of a supermassive black hole. Adam’s always fascinating musings can be followed on his Crowlspace site.
by Adam Crowl

The European Southern Observatory’s (ESO) GRAVITY instrument is a beam combiner in the infra-red K-band that operates as a part of the Very Large Telescope Interferometer, combining infra-red light received by four different telescopes, out of the eight operated (four 8.2 metre fixed telescopes and four 1.8 metre movable telescopes).
The latest measurements of the stars orbiting the Milky Way’s Galactic Core Super-Massive Black Hole (SMBH), otherwise known as Sagittarius A* (pronounced as ‘Sagittarius A Star’), by the GRAVITY instrument have determined its mass and distance to new levels of accuracy:
Ro = 8,275 parsecs (+\-) 9.3 parsecs and a mass of (in 106 Msol) 4.297 +\- 0.013.

Image: The galactic centre in infrared. Credit: NASA.
In round figures, that’s 27,000 light-years and 4.3 million Solar masses. The closest that light can approach a Black Hole and still escape is the Event Horizon, which is the spherical boundary at the distance of the Schwarzschild Radius, which is a radius of 2.95325 kilometres per solar mass. Thus 4.3 million solar masses is wrapped in an Event Horizon 12.7 million kilometres in radius. In aeons to come, when the Milky Way and M31 have collided and their black holes have coalesced, the combined Super Massive Black Hole (SMBH) will mass 100 million solar masses with an event horizon almost 300 million kilometres in radius.

Image: From Tales of Mystery and Imagination, by Edgar Allan Poe, with illustrations by Arthur Rackham (1935).
Into the Maelström
Mass-energy, so General Relativity tells us, puts dents into Space-time. Most concentrations of mass-energy, like stars, planets and galaxies, form shallow dents. Black Holes – like the future SMBH – go deeper, forming an inescapable waterfall of space-time inwards to their centres, the edge of which is the Event Horizon.
Light follows the curvature of space-time, traveling the shortest pathways (geodesics). At the Event Horizon the available geodesics all point towards the “middle” of the Black Hole. For particles with rest mass, like atoms, dust and space-ships, geodesics can’t be followed, merely approached, so they follow different pathways just as inexorably towards the centre.
Instead of flying radially inwards towards the future SMBH’s Centre, let’s ponder orbiting it. For most orbital distances from any Black Hole any small mass in orbit will experience nothing different to orbiting around any other large mass. Too close and you’ll experience extreme tidal forces if the black hole is small, so to avoid being torn to shreds when approaching really close a really big Black Hole is needed. The future SMBH massing 100 million solar masses with a Schwarzschild radius of 300 million kilometres has very mild Tidal Forces at the Event Horizon, though potentially significant for things as big as stars and planets.
We have multi-year ‘movies’ of stars orbiting around our SMBH, though none as close as we will explore. Close orbits get measured in multiples of M – which is half the Schwarzschild radius. At a radius of r = 3M space-time is so curved that the geodesics form a circle around the Black Hole. Light can thus orbit indefinitely, building up to potentially extraordinary energy densities if nothing else gets in its way, forming a so-called Photon Sphere. But the centre of the Galaxy is full of dust and gas, so something is always getting in the way. Eventually even photons get so energetic they perturb each other out of the Sphere.
For particles that don’t follow geodesics, merely approximate them, the Innermost Stable Circular Orbit (ISCO) is further out, at r = 6M. Objects here travel at half the speed of light. Other shapes of orbits can dip a bit closer in, down to r = 4M. Deeper in and motion near the Black Hole is no longer “orbital”. You must point away from the Black Hole and apply thrust or in-fall is inevitable.
The equation of orbital motion from the ISCO radius (rI) all the way to the centre was only recently worked out in closed form for rotating and non-rotating (stationary) Black Holes. Previously numerical Relativity methods were used, complicating modelling of Accretion Disks around astrophysical Black Holes. The Equation of Motion of a test particle (i.e. very small mass) around a non-rotating Black Hole, which our future SMBH might approximate, is straightforward:
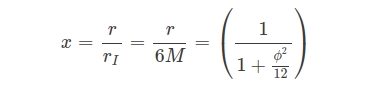
? is the angular distance traveled, with a range from negative infinity to zero, by convention. I’ve plotted r against ? here:
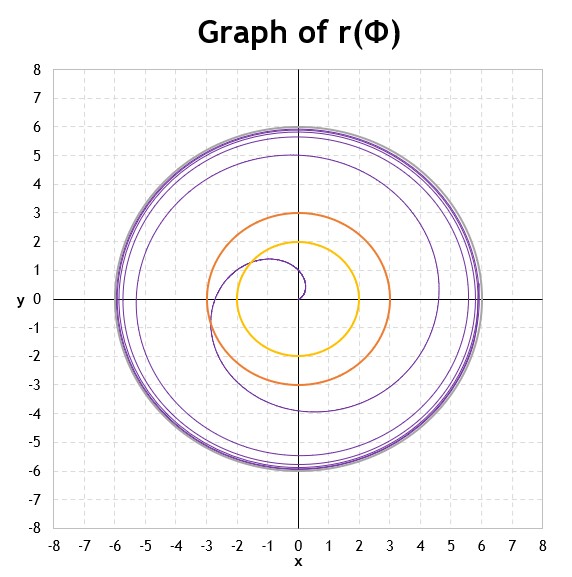
The Red Circle at r = 3 M is the Photon Sphere and the Yellow Circle at r = 2M is the Schwarzschild Radius aka Event Horizon. In this case the plot starts at r = 5.95M with the test particle circling the Black Hole 6 times before hitting the central point. The proper time experienced by an observer spiralling into the Centre is a bit more complicated. We can parameterise x as follows to make the mathematics easier:
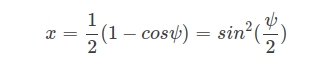
with ? running from an angle ? to 0. Then the Proper-Time ? of the inspiral trajectory is:
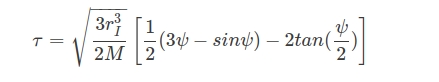
The above equation is true for any black-hole, spinning or stationary. For a stationary Black Hole, rI = 6M, so the equation simplifies to:
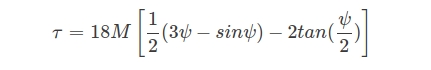
But what is M? It’s the “geometrised” mass of the Black Hole, which is derived by muliplying the mass by G/c2. Similarly the proper time is in units of “geometrised” time, so it needs to be divided by the speed of light, c, to convert to seconds.
In the case of the fall from r = 5.95M to r = 0, thus ? = (5.95/6) ? (?) to ? = 0, the total time is ? = 1291.14M. In the case of our Galaxy’s SMBH a proper time of M is 493 seconds. So the inspiral time is 176.6 hours and the Event Horizon is reached with 1.32 hours to go.
Surviving the Plunge
Falling into a Black Hole is probably fatal. However, like any fall, it’s not gravity that’ll kill you, but the sudden stop at the end. The final destination is the concentration of mass at the very centre. As the Centre is approached the first derivative of gravitational acceleration with respect to the radial distance vectors – the tidal forces – that will be experienced will become extreme.
Black holes are the pointy end of a spectrum of astrophysical objects. Stars exist due to their dynamic balance between the outward pressure from their fusion energy production and the inward pressure from their self gravity. When fusion energy production ends, the cores of stars begin collapsing, held above the Abyss of gravitational collapse by successive fusion energy reactions, then electron degeneracy pressure from squeezing free electrons too close together (via the Pauli Exclusion Principle), and when that isn’t enough, neutron degeneracy pressure and beyond.
Pressure is a measure of the ‘expansive’ energy packed in a volume. Dimensionally we can see that F / m2 (Pressure) = E / m3 (Energy per unit volume), so that as the mutual gravitational squeeze pushes inwards on a mass of particles which are pushing back against each other thanks to the Pauli Exclusion Principle for fermions (electrons, protons, neutrons etc) that pressure increases and increases, in a feed-back loop. Too much and equilibrium is never achieved. Thanks to Special Relativity we know that energy has mass, so that Pressure adds to the inward squeeze of gravity as particles are squeezed harder together. When the Gravity Squeeze – Push-Back Pressure process self-amplifies and runs away, the mass collapses ‘infinitely’ inwards forming a Singularity. Such a Singularity cuts itself of from the rest of the Universe when it squeezes inwards past the mass’s Schwarzschild Radius:
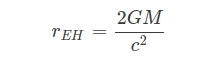
The resulting Event Horizon defines a Black Hole, by being a ‘surface of no return’ for everything, including light. Nothing escapes from within the Event Horizon. The minimum mass to cause such an inwards collapse and form a Black Hole for a mass of fermions (i.e. the same particles that make Stars, humans and space-ships) in the present day Universe is about 3 solar masses, squished into a volume smaller than 18 kilometres across.
Before we get to that point there are White Dwarfs and Neutron Stars – objects supported against collapse by Electron Degeneracy Pressure and Neutron Degeneracy Pressure, respectively. White Dwarfs are typically composed of carbon and oxygen – the ashes of helium fusion – and have observed masses anywhere between 0.1 and 1.3 Solar masses. Their radius is proportional to the inverse 1/3 power of their mass:
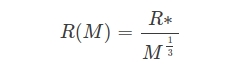
R* is a reference radius. For a cool white dwarf of 1 solar mass, the radius is about 0.8 Earth’s – 5,600 km. A space vehicle falling from infinity, on a flyby very close to such a star’s surface will rush past the lowest point of its orbit at 6,900 km/s, experiencing over 430,000 gee acceleration. In free-fall however it feels only the first derivative of that acceleration:
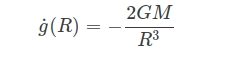
Which in this example is 0.15 gee per metre of radial stretching directed outwards and inwards along the direction of the radial distance to the white dwarf and a squeezing force half that directed laterally inwards from the sides. Easily resisted by small structures, like bodies and space-ships.
Neutron stars are smaller again – typically 20 km wide for a 1.3 solar mass neutron star. A near surface flyby isn’t recommended, since the tidal forces are thus almost a million times stronger. Close proximity to a magnetic neutron star is probably lethal anyway due to the intense magnetic fields long before the tidal forces rip you to shreds. Heavier neutron stars get smaller – just like white dwarfs – until they totally collapse as a black hole.
Black holes reverse the trend. The Event Horizon gets bigger linearly with their mass and there’s no upper limit to their mass. Our future Galactic SMBH’s Event Horizon will be 295.325 million kilometres in radius, give or take. Substituting the Schwarzschild Radius equation into the Tidal force equation gives us:
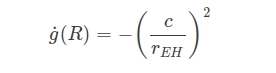
So the tidal force at the Event Horizon is 0.1 microgee per metre. The Moon could almost enter the Event Horizon peacefully…

How far into the SMBH can we, as Observers, then fall? If we can brace ourselves against 1,000 gees per metre of squeezing and stretching, then quite a long way…
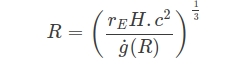
Which gives a distance of 139,430 kilometres from the centre. In other words 99.953% of the way to the central Singularity.
What wonders might we see? Quantum Gravity is yet to give a clear answer. Traditionally an imploding mass ends in the Singularity, which is a geometrical point. But quantum particles can’t be reduced to a singular point and retain quantum information. A possibility, due to the massively distorted space-time around the collapsing mass, is that ultimately the quantum particles all “bounce” after hitting Planck density and explode back outwards. To external Observers this is seen, in time-dilated fashion, as the slow-leak from the Event Horizon that is Hawking Radiation. Or, if the particles “twist” in a higher dimension, so they bounce as a new Big Bang forming another Universe. This can be seen as an emergence from a White Hole, as White Holes must keep expanding else they collapse into another Black Hole.
None of those options are ‘healthy’ to be around as flesh-and-blood Observer, so presently surviving the plunge is in doubt.
As we conclude, let’s check back in with Edgar Allan Poe, who knew a few things about terrifying plunges himself. In “Descent into the Maelstrom,” he gives us a look into what might be considered a 19th Century conception of a black hole and the journey into its bizarre interior:

“Looking about me upon the wide waste of liquid ebony on which we were thus borne, I perceived that our boat was not the only object in the embrace of the whirl. Both above and below us were visible fragments of vessels, large masses of building timber and trunks of trees, with many smaller articles, such as pieces of house furniture, broken boxes, barrels and staves. I have already described the unnatural curiosity which had taken the place of my original terrors. It appeared to grow upon me as I drew nearer and nearer to my dreadful doom. I now began to watch, with a strange interest, the numerous things that floated in our company. I must have been delirious — for I even sought amusement in speculating upon the relative velocities of their several descents toward the foam below. ‘This fir tree,’ I found myself at one time saying, ‘will certainly be the next thing that takes the awful plunge and disappears,’ — and then I was disappointed to find that the wreck of a Dutch merchant ship overtook it and went down before. At length, after making several guesses of this nature, and being deceived in all — this fact — the fact of my invariable miscalculation — set me upon a train of reflection that made my limbs again tremble, and my heart beat heavily once more.”
References
Mummery, A. & Balbus, S. “Inspirals from the innermost stable circular orbit of Kerr black holes: Exact solutions and universal radial flow,” Physical Review Letters 129, 161101 (12 October 2022).
https://doi.org/10.48550/arXiv.2209.03579
Fragione, G. and Loeb, A., “An Upper Limit on the Spin of SgrA* Based on Stellar Orbits in Its Vicinity” (2020) ApJL 901 L32
DOI 10.3847/2041-8213/abb9b4



“Falling into a Black Hole is probably fatal. However, like any fall, it’s not gravity that’ll kill you, but the sudden stop at the end. The final destination is the concentration of mass at the very centre.”
I can’t be mass at the centre but more than likely field energy i.e. energy stored in the very fields that make up the fabric of the universe.
What I found interesting is that apparently one could fall inside the event horizon of a SMBH as the gravity field gradient was so low, unlike regular BH where the high gradient “spaghettifies” objects. So a probe could be sent into the SMBH and successfully enter the BH. As the outside observers lost contact, the probe would note that the external universe of stars would also disappear. What would it see inside the EH? I can imagine that any body emitting light would have the rays bent by the gravitational fields so that an observerser might see the light coming from everywhere – ie inside the EH it would appear to be lit by any light-emitting object rather than seeing the object as a light source.
That is not quite true because the observer can’t stay at a fix point and receive the light from all directions. The observer has to “fall” with everything else including the light around it, that put a very strong constraint on what the observer can actually “see”.
Due to time dilation, wouldn’t the probe witness the death of the blackhole as well as the external universe disappear?
There are simulations on youtube about falling into a BH, where you don’t actually notice crossing the event horizon. It still appears to be “under” you, and stars are still visible but more and more warped as you descend.
Can you explain how hawking radiation escapes from a BH? My understanding is that particles can form in space near the event horizon (EH), where some are just outside the EH. So far so good. But unless they are orbiting the BH at near c, the particles will be pulled back inside the EH and there will be no observable Hawking radiation and therefore no evaporation of the BH. So these particles can only be photons to escape. Is this correct, or have I got something terribly wrong in my understanding?
If they are photons, will the gravitational forces redshift these photons, and if so, is there a formula that will predict their wavelength?
“Can you explain how hawking radiation escapes from a BH? ”
are u, Alex Tolley , asking this ques. or are u being rhetorical ? If asking, I think the ans. is that the particles form in space where they are just outside the EH, and that they ARE orbiting the BH at c .
The way Hawking radiation is described in media is based on a dumb-downed description made by Hawking that has little to do with how Hawking radiation works.
https://www.youtube.com/watch?v=rrUvLlrvgxQ
Hi Alex
That’s a very good question which confuses even physicists. The curious thing about Hawking Radiation, which might help explain how it escapes, is that the particles (which are field excitations in Quantum Field Theory) are emitted at a certain temperature. As Wien’s Law tells us, the inverse of the temperature determines the wavelength of peak emission. The Hawking Temperature is so low that the wavelength is on average much bigger than the Black Hole’s Event Horizon. Thus its emission isn’t really that close to the Event Horizon and in one sense it’s already “red-shifted”.
Another aspect to high-light is that an infalling Observer doesn’t observe Hawking Radiation coming from the Event Horizon.
Studies of Quantum Field Theory on the other side of the Event Horizon show that the energy density of the Hawking Radiation increases all the way up to the Singularity, proportional to the sixth-power of the inverse radial distance to the Singularity. So it gets very HOT inside, in a sense because Space-time is being “compacted” as the Singularity is being approached.
An important caveat is that present theories of what happens to the collapsing non-quantum “mass-energy” that forms a Singularity in Classical General Relativity has to leave out aspects of particle physics that might become important. General Relativity can’t handle the instrinsic “spin” of particles, unless new mathematical assumptions are made. One such extension is General Relativity plus Torsion (intrinsic “twistiness” of space-time), which causes imploding particles-with-spin to bounce into a new White Hole Big Bang.
Until the alternative particle physics used to study collapsing matter is verified experimentally, via observation or replication via physical analogues, it remains speculative. Neutron stars are objects on the verge of collapse so their properties are key to getting hints of how real matter behaves in the extremes of collapse.
I’ve seen other explanations such as quantum tunneling from the event horizon, and there is also quite a bit of ongoing research into “black hole hair” that is surely much more informed that what I’m about to say.
But I think the most important way to answer this question is in terms of the Unruh effect. Staying out of a black hole means accelerating. Any observer in an accelerating frame of reference will detect radiation with a temperature of (h/c)(a/kB), which is to say an average thermal energy of (h/c)a. Now if we consider a simple black hole with radius GM/c2 and use the classical law of gravitation (F = GmM/r2, divided by m), the acceleration of an object at the event horizon will be c4/GM. Plugging that into the equation above, I get E = hc3/GM. Now this is 4 times more than the actual equation for black hole temperature I looked up, perhaps because I’m using Newtonian physics on a black hole?, but at least the form is the same.
The boggle here is why do we (hypothetically) detect phantom particles when we are accelerating, which don’t exist at rest? Where do they “come” from? I don’t pretend to understand that. I wonder if it is related to Tkatchenko’s recent paper that cracks the mystery of dark energy (follow links from the write-up): https://phys.org/news/2023-01-approach-mystery-dark-energy.html There the vacuum is viewed as full of virtual particles which give it a finite polarizability. I think that means that space sticks together something like molecules of oil are held together by dispersion forces.
Thank you for that link.
Apparently, the author of that video had the same questions as I did, making me feel relieved I have company. :-)
While I am not sure that I understand why thinking about the particles is like pinching strings, the conclusion seems to bear out my thought and makes the wavelength question relevant.:
1. the particles are mostly photons.
2. The wavelength is in the ultra long wave radio spectrum. [If the wavelength is the diameter of the BH, that would make the photons appearing from a smbh as really, really, loooooooooonnnnnngggggggg.]
(so they answer both my questions)
The useful part was understanding that the photons can appear well away from the EH and therefore not be drawn back into the BH.
Hi Alex
If you want the hard details here’s a good reference:
Sparsity of the Hawking flux
Basically the wavelength of peak emission of Hawking radiation is almost 80 times (8*pi^2) the Schwarzschild Radius.
Would putting a telescope in orbit around one of these objects be useful? Putting it another way, would creating a BH at will, be a way to help see far away locales/destinations?
Completely OT and off my area of knowledge.
Sorry for breathing life into a dead end of an idea but…
….would it be possible to create microscopic (or slightly bigger) BHs to increase the magnification power of telescopes – or at least factor it into the design of a telescope.
You could create microscope BHs within a field and focus in on that point to “capture” an opportune moment.
This paper does not differentiate black holes from singularities which is a common misconception. Some astrophysicists still go along with that idea. The singularity is how our universe was postulated to have started and the white hole on the other side of it was supposed to be a passage into another universe. Sagittarius A is not a singularity.
I am biased against the idea of an event horizon in black holes. I intuit that the black star model of black holes is correct which includes a black star with a very hot center, but no event horizon, but with may layers like an onion with each layer closer to the center denser than the surface All black holes would be black stars composed only of degenerate matter like a Neutron stars, but the difference is that once the Paulit exclusion principle between the neutrons is over powered, we get a quark gluon plasma or some kind of degenerate matter, but not a bottomless pit or hole in space time. Energy and matter at the center of a black hole would not go faster than the speed of light, so therefore it would not violate general and special relativity since any particles there would not be able to reach the speed of light but be very close to the speed of light like in the LHC. Also one can’t start out with the finite energy of a star and end up with infinite energy or density. An escape velocity faster than light is not infinite. Special relativity prevents all matter particles an from reaching the speed of light. Energy is limited to the speed of light. Source, the paper: Black Stars, not Holes, Scientific American, October 2009, pages 39 to 45. https://physics.ucf.edu/~britt/AST2002/R9-Barcelo-Black%20stars,%20not%20holes.pdf
Hi Geoffrey,
Roy Kerr, who solved the Space-time for rotating black holes, is something of a sceptic about speculations about what’s beyond the Event Horizon. But it’s a common misconception about escape velocity inside a Black Hole as one approaches the central mass – it does exceed the speed of light because Space-time itself is infalling into the centre. There’s no limit on the speed of Space-time, and that’s General Relativity.
Quote by Adam Crowl: But it’s a common misconception about escape velocity inside a Black Hole as one approaches the central mass – it does exceed the speed of light because Space-time itself is infalling into the centre. There’s no limit on the speed of Space-time, and that’s General Relativity.” We agree that space can move faster than light which makes a warp drive possible and the expansion of space. It’s a different story when it comes to gravity waves which according to general relativity are limited to the speed of light. The problem with the event horizon idea is that all the four forces including gravity waves are limited to the speed of light according to special relativity. If an event horizon had an escape velocity faster than the speed of light, then even gravity could not escape it and we would have no way of correctly measuring the mass of a black hole, but we can by the stars which orbit close to Sagittarius A, so consequently, the event horizon idea must be invalid.
With the black star model, I do think there might be more stopping the black hole from completely collapsing like the radiation pressure from quark particles and extremely high energy gamma rays which are emitted by the vibration and rotation of quarks caused by the extremely strong gravitational wave particles in the center of the core where it must be very hot. There may be a Pauli exclusion principle between the quarks or maybe even a quark condensate? The premise is that with the knowledge we already know in physics we can predict what is in the center of a black star.
How gravity escapes from a black hole is a common forum question, usually linked to the question of how an electric field escapes a black hole. There are unusual answers, such as that virtual particles can go FTL and pass through the event horizon ( https://facultystaff.richmond.edu/~ebunn/ajpans/ajpans.html ). A more typical answer is simply that an outside observer never actually sees the last photon (or graviton, I suppose) from an infalling object, because the emissions are infinitely redshifted; from your frame of reference the objects are still “there” even if it is impossible in practice to detect them. I think another appealing explanation is that the gravitational and electric _fields_ are real things exist in space on their own. By that thinking the source particles represent fluctuations that can change the fields by moving, but perhaps they don’t need to be in constant communication by virtual FTL particle to stop the field from suddenly going away.
Hi Geoffrey
It’s true that gravitational waves (not gravity waves, which are a different thing entirely) travel at light-speed. And completely irrelevant. The field of the collapsing mass of the Black Hole remains even after the Event Horizon forms. Nothing that happens to the collapsing mass past that point can be communicated with the external Universe – it has become a “frozen star” to coin an old phrase. The field remains what it was at the moment of Event Horizon creation.
Just about every sentence of this comment is wrong, a rejection of well tested evidence and theory, or an assertion of bald speculation as fact. To be blunt, it isn’t worth a response. But I expect there will be a few!
Hey, he did give a source. Wacky ideas about black holes are certainly nothing new. Pedantically I could say if space beyond the event horizon is unobservable, then talking about what happens there is not even science; there would be no right and wrong, only “interpretation” as with QM models. The cited article shares an etiology with Tipler’s archived Geocities page someone posted recently – the black hole information paradox seemed very strong in 2009, and was powering unusually creative thinking. Nothing written there bothers me as much as the popular Penrose-Carter diagrams still sometimes used to claim that objects travel via rotating black holes to other universes… even though the black hole would have to survive for an infinite time for anything to get there on the diagram. Let’s celebrate creativity, debate, learning … and more up-to-date links to reference for our pet alt-hypotheses when possible. :)
If gravity can’t escape the event horizon, then most of the mass would disappear from our measurable universe. Furthermore there could not be any mass outside the event horizon moving at less the light speed because it would be sucked into the black hole. One has to stick to the principles of astrophysics here, that we can measure the mass of the black hole using general relativity; the speed of the stars orbiting around Sagittarius A are completely supported by GE and give us that mass. Quote from Astrophysics Spectator: “The mass of Sgr A* can then be derived directly from the velocity and radius through the equation relating centrifugal force to the gravitational force: G Mbh = V2r, where G is the gravitational constant and Mbh is the mass of the black hole. In this way both the distance and the mass of Sgr A* are found.” This cannot be ignored. The event horizon idea is only theory, but not fact and is an idea that was not challenged until the Black Stars, not holes paper which intrigued me when in read it in 2012.
In physics, one’s theory has to more than just match observations, but has to conform to first principles like General relativity which is a force that controls the large scales. If the theory does not conform to it, then it must be wrong which is how physicists view first principles which are impersonal.
I think talking about the conditions inside black holes is like discussing the plays Shakespeare might have written had he not died when he did.
We can observe black holes from a distance, and collect data about what is happening in their vicinity, but we can never learn what is happening inside.
It shouldn’t be all that difficult to adjust to this. After all, we don’t know where we go after we die, and we don’t know where we were before we were conceived. Not only do we not know the answer, it may not even be a proper question.
I think you are conflating religion with physics. How and where one dies does not matter, but only what happens afterwards. Quantum field theory and atomic physics has allowed us to understand neutron stars and we can’t see what is in them in the visible spectrum, but observations and high energy particle physics has allowed us to know they are made of neutrons with a crust of electrons which are separated out of the neutrons by the centrifugal force and flung off into space.
There can be another reason why we don’t see any electromagnetic radiation from a black star. They symbolize death because if you fall into one you will be crushed smaller than an atom. Since a black hole is made up of degenerate matter denser than a neutron star, and both of these do not have any chemical elements in them, but nothing but neutrons and degenerate matter. Consequently without chemical elements there never can be any nuclear fusion as in ordinary stars, so therefore degenerate matter does not emit any electromagnetic radiation which includes the entire EM spectrum. Consequently, black holes are dead stars which are dark stars invisible to the electromagnetic spectrum. Degenerate matter is also solid, so it blocks light in the visible spectrum and all EMR or does not emit any EMR, not because there is a mythical event horizon which has an escape velocity greater than light.
Did you know that black holes also twinkle?
https://theconversation.com/why-do-black-holes-twinkle-we-studied-5-000-star-eating-behemoths-to-find-out-198996
The objects orbiting this behemoth may be of the greatest interest-small black holes about the center might centrifuge matter caught in between…in a zone where the two BHs cancel out-a jet could be born. The galaxy center should be the first solar foci ‘scope object-then Andromeda.
Black Holes are indeed strange creatures not only can you see near the beginning of time by its magnification powers but also the end of time by merely looking at it.
The emission of invisible, thermal black body radiation is the result of the vibration and rotation of atoms and molecules, but what if there are not any of these, but only degenerate matter which might behave differently when it is hot, i.e., not emit any EMR.
Have a look at this: https://phys.libretexts.org/Bookshelves/
Relativity/General_Relativity_(Crowell)/
06%3A_Vacuum_Solutions/6.01%3A_Event_Horizons (copy and paste the link – I added some returns in the middle of this long link so it doesn’t derange the website’s CSS)
If you are in an ordinary spaceship in ordinary flat space, and accelerate, there is an appearance of an event horizon in space behind you, because light emerging from far enough back won’t catch up to you, ever, unless you stop accelerating. And that event horizon is associated with Unruh radiation just like Hawking radiation from a black hole.
Geoffrey,
If degenerate matter doesn’t emit EMR then planets and stars would be more inclined to implode due to gravity. EMR is what keeps them suspended against their own gravity. EMR lets hot matter exchange heat and it inflates stars against their own mass via the pressure thus created. There is no other force to keep the non-degenerate parts of a star from imploding onto the degenerate core.
Every moving charge that is accelerated *must* produce EMR. That’s basic physics. It’s a fundamental property of charged particles that can’t be conveniently be waved away when it’s needed by some ‘theory’. Degenerate matter is ionised – it’s all free electrons and their ions – so every constituent is a potential emitter of EMR. The Pauli Exclusion Principle which creates degeneracy pressure relies on those free electrons being squeezed together to create it. No free charges, no Degenerate Matter.
Maybe you’re thinking of Neutron Degenerate Matter? I hinted that its still largely unknown properties might actually keep astrophysical objects suspended against further collapse after they form an Event Horizon, but the analysis by Tolman–Oppenheimer–Volkoff is yet to be refuted, even if it has been updated by modern particle physics.
If exotic matter could resist the forward flow of time toward the singularity in a black hole, it might show similar effects outside, and be usable for FTL communication. It’d be interesting…
Quote by Adam Crowl: “If degenerate matter doesn’t emit EMR then planets and stars would be more inclined to implode due to gravity.” Well, this statement is beside my point and I agree with it. I am not saying that all degenerate matter does not emit EMR which is an extreme position I don’t hold. Also you make an important point that that astrophysicists are not that confident in physics of neutrons stars, the forces between the neutrons inside them. Some say that the strong nuclear force is both attractive and is repulsive there. In fusion in stars, the electromagnetic force or proton repulsion is over come when the energy of the protons is high enough and the two protons get close enough to fuse and energy is released. This is never done directly though because the speed of the protons is never fast enough and therefore not hot enough even in the fusion in the hottest stars, so like the our Sun, fusion has to happen indirectly through the weak nuclear force and proton proton chain. In the first part of the proton proton chain, two protons collide and one of them turns into a neutron when the positive charge of the proton is carried away by a positron and an neutrino. This is where the strong nuclear force works.
In a neutron star, the electromagnetic force and the photon the gauge boson or carrier of the electromagnetic force gets over powered. The electrons are forced into the protons and they become neutrons. Neutrons are called that because they are charge neutral, there is no electric charge, so some other force must be repelling them, the strong nuclear force. Also the Pauli exclusion principle between the neutrons keeps them apart. Since there are no electric forces and electrons, the size of the neutron star is much smaller and denser than a white dwarf. A black hole is assumed to be even smaller and denser than neutron star because the Paulit exclusion principles between the neutrons is overcome. The physics of what happens after this is unknown. By process of elimination we can rule some things out like a bottomless pit in space when the foundation of matter collapses, an assumption that has not been proven. One thing is for certain that it is physically impossible for a black hole to emit gravity waves at the speed of light, but have an event horizon that is has an escape velocity faster than light since gravity cant escape. Neutron stars are bright in the x ray spectrum. I would like to see some more sensitive tests in the x rays of Sagittarius A to see if the main body of the black hole might emit x rays as well as the accretion disk. This would prove that black hole degenerate matter emits EMR. Even if it does not does not mean that it has an event horizon with an escape velocity faster than light. There might be some other reason we have not discovered yet. I will admit that this needs further research, but from a principled view GR, the FTL event horizon idea looks like more theory than fact.
Before fusion in stars can occur, the protons have to get close enough “for the strong nuclear force to grab them.” The temperature 10 million Kelvin. The pressure in the center of the Sun 265 billion bars or Earth atmospheres.
Relativistic orbits around black holes seem to get a little complicated. :) Apsidal precession can turn an orbit into a four-leaf clover, or stranger. (I think I remember reading somewhere even an Earth-like planet with zero eccentricity wouldn’t really be perfectly circular if you could look precisely enough, but some manner of cycloid?)
https://cr4.globalspec.com/blogentry/5832/Interesting-Relativistic-Orbit-2
An exclusion principle which applies to gravity could exist preventing a singularity. Under immense concentrations of matter and energy the gravity field could change or its interactions with the other fields when they unify.
Quote by Michael: “An exclusion principle which applies to gravity could exist preventing a singularity.” This principle is called special relativity which states that particles with mass can’t ever reach the speed of light because an infinite amount of energy is needed. It does not mean that they can’t get really close to the speed of light like in the LHC. The temperature of the collision is 7.2 trillion degrees based on the kinetic energy of the debris particles. I imagine the center of a black hole is hotter.
More like the Plank temperature
1.416784(16)×10^32 K