Centauri Dreams
Imagining and Planning Interstellar Exploration
Holiday Thoughts on Deep Time
An old pal from high school mentioned in an email the other day that he had an interest in Adam Frank’s work, which we’ve looked at in these pages a number of times. Although my most recent post on Frank involves a 2022 paper on technosignatures written with Penn State’s Jason Wright, my friend was most intrigued by a fascinating 2018 paper Frank wrote for the International Journal of Astrobiology (citation below). The correspondence triggered thoughts of other, much earlier scientists, particularly of Charles Lyell’s Principles of Geology (1830-1833), which did so much to introduce the concept of ‘deep time’ to Europe and played a role in Darwin’s work. Let’s look at both authors, with a nod as well to James Hutton, who largely originated the concept of deep time in the 18th Century.
Adam Frank is an astrophysicist at the University of Rochester, and one of those indispensable figures who meshes his scientific specialization (stellar evolution) with a broader view that encompasses physics, cultural change and their interplay in scientific discourse. He fits into a niche of what I think of as ‘big picture’ thinkers,’ scientists who draw out speculation to the largest scales and ponder the implications of what we do and do not know about astrophysics for a species that may spread into the cosmos.

Now in the case of my friend’s interest, the picture is indeed big. Frank’s 2018 paper asked whether our civilization is the first to emerge on Earth. Thus the ‘Silurian’ hypothesis, explored on TV’s Doctor Who in reference to a race of intelligent reptiles by that name who are accidentally awakened. The theme pops up occasionally in science fiction, though perhaps less often that one might expect. James Hogan’s 1977 novel Inherit the Stars, for example, posits evidence for unknown technologies discovered on the Moon that apparently have their origin in an earlier geological era.
Image: Astrophysicist Adam Frank. Credit: University of Rochester.
I won’t go through this paper closely because I’ve written it up before (see Civilization before Homo Sapiens?), but this morning I want to reflect on the implications of the question. For it turns out that if, say, a species of dinosaur had evolved to the point of creating technologies and an industrial civilization, finding evidence of it would be an extremely difficult thing. So much so that I find myself reflecting on deep time in much the same way that I reflect on the physical cosmos and its seemingly endless reach.
Consider that we can trace our species back in the Quaternary (covering the last 2.6 million years or so) and find evidence of non-Homo Sapiens cultures, among which the Neanderthals are the most famous, along with the Denisovians. Bipedal hominids show up at least as far back as the Laetoli footprints in Tanzania, which date to 3.7 million years ago and were apparently produced by Australopithecus afarensis. Frank and co-author Gavin Schmidt also note that the largest ancient surface still available for study on our planet is in the Negev Desert, dating back about 1.8 million years.
These are impressive numbers until we put them into context. The Earth is some 4.5 billion years old, and complex life on land has existed for about 400 million of those years. Let’s also keep in mind that agriculture emerged perhaps 12,000 years ago in the Fertile Crescent, and in terms of industrial technologies, we’ve only been active for about 300 years (the authors date this from the beginning of mass production methods). Tiny slivers of time, in other words, amidst immense timeframes.
So as Frank and Schmidt point out, we’re talking about fractions of fractions here. There is a fraction of life that gets fossilized, which in all cases is tiny and also varies according to tissue, bone structure, shells and so forth, and also varies from an extremely low rate in tropical environments to a higher rate in dry conditions or river systems. The dinosaurs were active on Earth for an enormous period of time, from the Triassic to the end-Cretaceous extinction event, something in the range of 165 million years. Yet only a few thousand near-complete dinosaur specimens exist for this entire time period. Would homo sapiens even show up in the future fossil record?
From the paper:
The likelihood of objects surviving and being discovered is similarly unlikely. Zalasiewicz (2009) speculates about preservation of objects or their forms, but the current area of urbanization is <1% of the Earth’s surface (Schneider et al., 2009), and exposed sections and drilling sites for pre-Quaternary surfaces are orders of magnitude less as fractions of the original surface. Note that even for early human technology, complex objects are very rarely found. For instance, the Antikythera Mechanism (ca. 205 BCE) is a unique object until the Renaissance. Despite impressive recent gains in the ability to detect the wider impacts of civilization on landscapes and ecosystems (Kidwell, 2015), we conclude that for potential civilizations older than about 4 Ma, the chances of finding direct evidence of their existence via objects or fossilized examples of their population is small.
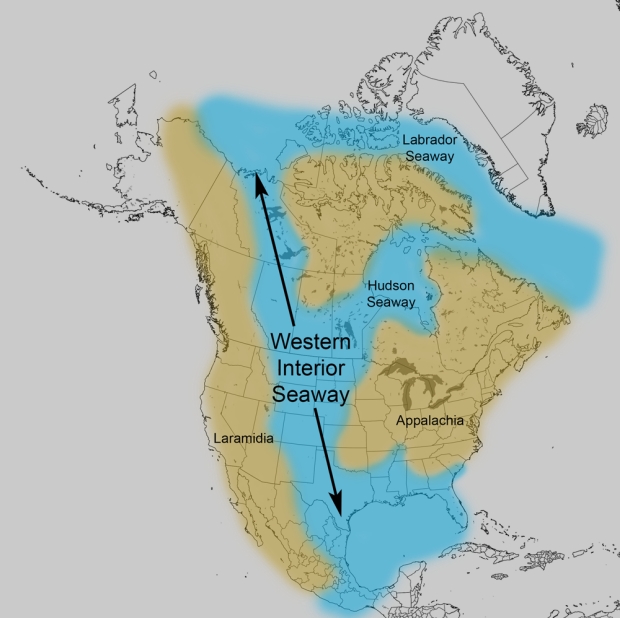
Image: The Cretaceous-aged rocks of the continental interior of the United States–from Texas to Montana–record a long geological history of this region being covered by a relatively shallow body of marine water called the Western Interior Seaway (WIS). The WIS divided North America in two during the end of the age of dinosaurs and connected the ancient Gulf of Mexico with the Arctic Ocean. Geologists have assigned the names “Laramidia” to western North America and “Appalachia” to eastern North America during this period of Earth’s history. If a species produced a civilization in this era, would we be able to find evidence of it? Credit; National Science Foundation (DBI 1645520). The Cretaceous Atlas of Ancient Life is one component of the overarching Digital Atlas of Ancient Life project. CC BY-NC-SA 4.0 DEED.
Intriguing stuff. The authors advocate exploring the persistence of industrial byproducts in ocean sediment environments, asking whether byproducts of common plastics or organic long-chain synthetics will be detectable on million-year timescales. They also propose a deeper dive into anomalies in current studies of sediments, the same sort of analysis that has been done, for example, in exploring the K-T boundary event but broadened to include the possibility of an earlier civilization. I send you to the paper, available in full text, for discussion of such testable hypotheses.
Back to deep time, though, and the analogy of looking ever deeper into the night sky. In asking how long a civilization can survive (Drake’s L term in the famous equation), we ask whether we are likely to find other civilizations given that over billion year periods, they may last only as a brief flicker in the night. We have no good idea of what the term L should be because we are the only civilization we know about. But if civilizations can emerge more than once on the same world, the numbers get a little more favorable, though still daunting. A given star may be circled by a planet which has seen several manifestations of technology, a greater chance for our detection.
A cycle of civilization growth and collapse might be mediated by fossil fuel availability and resulting climate change, which in turn could feed changes in ocean oxygen levels. Frank has speculated that such changes could trigger the conditions for creating more fossil fuels, so that the demise of one culture actually feeds the energy possibilities of the next after many a geological era. How biospheres evolve – how indeed they have evolved on our own world – is a question that exoplanet research may help to answer, for we have no shortage of available worlds to examine as our biosignature technologies develop.
Culturally, we must come to grips with these things. In an essay for The Geological Society, British paleontologist Richard Fortey discusses the seminal work of James Hutton and Charles Lyell in the 18th and 19th Centuries in developing the concept of geological time, which John McPhee would present wonderfully in his 1981 book Basin and Range (I remember reading excerpts in The New Yorker). The Scot James Hutton had literary ambitions, publishing his Theory of the Earth in 1795 and changing our conception of time forever. Hutton knew Adam Smith and spent time with David Hume; he would also have been aware of French antecedents to his ideas. But despite its importance, even Lyell would admit that he found Hutton’s book all but unreadable.
It took a friend named John Playfair to turn Hutton’s somnolent prose into the simplified but clear Illustrations of the Huttonian Theory of the Earth in 1802, making the idea of deep time available to a large audience and leading to Lyell. Which goes to show that sometimes it takes a careful popularizer to gain for a scientist the traction his or her work deserves. The emphasis there is on ‘careful.’
Lyell’s Principles of Geology, published in three volumes between 1830 and 1833, famously traveled with Darwin on the Beagle and, as Fortey says, “donated the time frame in which evolution could operate.” He goes on:
“…once the time barrier had been breached, it was only a question of how much time. The stratigraphical divisions of the geological column, the periods such as Devonian or Cambrian, with which we are now so familiar, were themselves being refined and put into the right sequence through the same historical period. Just to have a sequence of labels helped geologists grapple with time, and, in a strange way, labels domesticate time.

But domestication co-exists with wonder. I imagine the most hardened geologist of our day occasionally quakes at the realization of what all those sedimentary layers point to, a chronological architecture — time’s edifice — in which our entire history as a species is but a glinting mote on a rockface of the future. Our brief window today is reminiscent of Hutton and Lyell’s. Like them, we are compelled to adjust to a cosmos that seems to somehow enlarge every time we probe it, inspired by new technologies that give birth to entire schools of philosophy.
John Playfair would write upon visiting Siccar Point, the promontory in Berwickshire that inspired Hutton’s ideas, that “The mind seemed to grow giddy looking so far into the abyss of time.” We are similarly dwarfed by the vistas of the Hubble Ultra Deep Field and the exquisite imagery from JWST. Who knows what we have yet to discover in Earth’s deep past?
The paper is Schmidt and Frank, “The Silurian Hypothesis: Would it be possible to detect an industrial civilization in the geological record?” published online by the International Journal of Astrobiology 16 April 2018 (full text). Gregory Benford’s Deep Time: How Humanity Communicates Across Millennia (Bard, 2001) is a valuable addition to this discourse. For a deeper dive, Fortey mentions Martin Rudwick’s Bursting the Limits of Time: The Reconstruction of Geohistory in the Age of Revolution ( University of Chicago Press, 2007). Fortey’s own Life: A Natural History of the First Four Billion Years of Life on Earth (Knopf, Doubleday 1999) is brilliant and seductively readable.

A Novel Strategy for Catching Up to an Interstellar Object
Reaching ‘Oumuamua through some kind of statite technology, an idea we’ve been kicking around recently, brings up the interesting work of Richard Linares at MIT, who has been working on a “dynamic orbital slingshot” for rendezvous with future objects from the interstellar depths (ISOs). Linares received a Phase I grant from the NASA Innovative Advanced Concepts (NIAC) Program to pursue the idea of a network of statites on sentry duty, any one of which could release the stored energy of the sail to enter a trajectory that would take it to a flyby of an object entering our system on a hyperbolic orbit.
The concept is simplicity itself, once you realize that a statite balances the pressure of solar photons against the Sun’s gravitational pull, and essentially hovers in place. As I mentioned when covering Greg Matloff and Les Johnson’s paper on using statites to achieve fast rectilinear trajectories to reach interstellar interlopers, Robert Forward was the one who came up with the idea and practical uses for it. He could envision, for example, communications satellites in polar position to cover high latitudes on Earth.
Here’s what Forward said about the statite concept in his wonderful essay collection Indistinguishable from Magic (1995):
…I have the patent on it — U.S. Patent 5,183,225 “Statite: Spacecraft That Utilizes Light Pressure and Method of Use”… The unique concept described in the patent is to attach a television broadcast or weather surveillance spacecraft to a large highly reflective lightsail, and place the spacecraft over the polar regions of the Earth with the sail tilted so the light pressure from the sunlight reflecting off the lightsail is exactly equal and opposite to the gravity pull of the Earth.
You can see why we need a new term here. If you deploy a sail in the configuration Forward describes, it essentially sits over the polar region while the Earth rotates below it. In other words, technically it is not a satellite. ‘Statite’ is a Forward coinage to describe such a hovering object in space. He wrote of a statite he dubbed the ‘Hovering Hawke’ in one of his short stories. It would be placed too far from the surface to be effective as a communications satellite, but could offer direct broadcasting to places on Earth that are without that capability. Weather surveillance is another use.
Polesitters become interesting when we consider the nature of a geostationary orbit. Put a satellite directly over the equator at 35,786 kilometers altitude and it will appear stationary over the Earth, a useful trait for communications. But the satellite must be positioned directly above the equator, matching Earth’s rotation, to maintain its position relative to the surface.

If we put our satellite at an angle relative to the equator, its apparent motion on Earth will be a figure eight, in what is called an inclined geosynchronous (not geostationary) orbit. That’s useful for areas not covered by geostationary satellites but not good enough for continuous coverage of a specific area, especially the more latitudinally challenged regions like the poles, and that’s why the polesitter is attractive. It can give us continuous coverage even when the region it sits above is far from the equator.
Image: Analog‘s December, 1990 issue contained an article by Robert Forward describing the ‘polesitter’ concept, one of many innovative ideas the scientist introduced to a broad audience. Credit: Condé Nast.
There’s always a catch, and here’s the catch with polesitters, as Forward explained it in his article. When the summer months arrive and the polar regions are in sunlight, keeping the statite precisely balanced (to maintain the hover) becomes quite tricky. He saw that such seasonal instability demanded that a statite be relatively far from Earth, and calculated that it cannot get any closer than 250 Earth radii to the surface.
But Linares and team are not thinking about statites supplying services to Earth. The NIAC work explores using statites to set up an early warning system for interstellar objects, one that will allow fast intercepts before these interlopers blow through our system and return to interstellar space. Consider what happens when we ‘turn off’ the statite capability on our satellite (as from rotating the sail to an edge-on position, for example, or simply releasing a CubeSat). At this point, the released object has no forces impinging upon it but gravity. Let me quote Linares from a white paper on the subject:
…a statite at 1 AU has a free-fall trajectory of about 64 days. This fast response time to a potential ISO can be thought of as a slingshot effect, since the solar sail is used to “store energy” that is released when desired. Additionally, to achieve a flyby some Delta-V is required to adjust from the free-fall path to a flyby trajectory. The proposed mission for the statite concept is to utilize a constellation of such devices to achieve wider coverage over a spherical region of 1 AU for potential ISO missions. Additionally, the orbital plane can be adjusted with relatively low Delta-V.
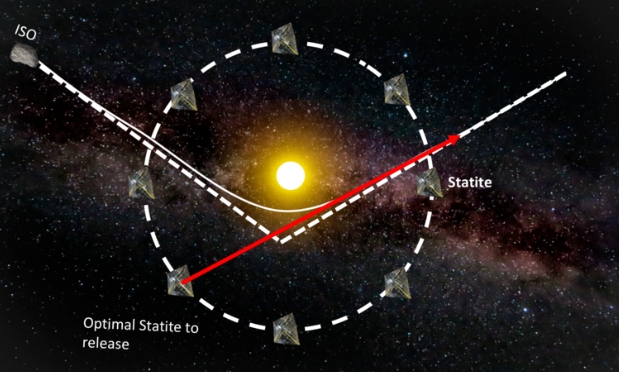
Image; A constellation of statites as envisioned by Richard Linares for intercepting a future interstellar interloper. Credit: MIT/Richard Linares.
The levitating sail has an inertial velocity of zero, and when released from ‘hover,’ it enters a Keplerian orbit. So as Linares points out, we can turn any one of the statites in our constellation of statites into a ‘sundiver,’ hurtling toward the Sun before its trajectory is adjusted by use of the sail (or perhaps other propulsion). Which statite is deployed simply depends upon the optimum trajectory to the incoming ISO.
We are now on a fast track toward reaching the interstellar object with at least a flyby. Linares calls this a “dynamic orbital slingshot for rendezvous with interstellar objects.” And the idea is to have a constellation of these statites always at the ready for the next ‘Oumuamua. Or, considering how odd ‘Oumuamua seems to be, perhaps I should say “the next Borisov.” Even so, with this net, who knows what we might catch?
The paper makes the case that a statite free-falling toward the Sun from an initial position at 1 AU and then deploying its sail away from the Sun at perihelion can achieve speeds of up to 25 AU/year, making it possible to deliver payloads to the outer Solar System. Now we’re in Matloff/Johnson ‘sundiver’ territory. Voyager 1 has reached 3.6 AU per year by comparison, making the statite concept attractive beyond its value as a station-keeper for quick response missions to interstellar comets/asteroids.
For more on Richard Linares’ work, see “Rendezvous Mission for Interstellar Objects Using a Solar Sail-based Statite Concept,” a white paper available on arXiv.

Forbidden Worlds? Theory Clashes with Observation
Back before we knew for sure there were planets around other stars, the universe seemed likely to be ordered. If planet formation was common, then we’d see systems more or less like our own, with rocky inner worlds and gas giants in outer orbits. And if planet formation was a fluke, we’d find few planets to study. All that has, of course, been turned on its head by the abundant discoveries of exoplanets galore. And our Solar System turns out to be anything but a model for the rest of the galaxy. In today’s essay, Don Wilkins looks at several recent discoveries that challenge planet formation theory. We can bet that the more we probe the Milky Way, the more we’ll find anomalies that challenge our preconceptions.
by Don Wilkins
The past few decades have not been easy on planet formation theories. Concepts formed on the antiquated Copernican speculation, the commonality of star systems identical to the Solar System, have given way to the strangeness and variety uncovered by Kepler, Hubble, and the other space borne telescopes. The richness of the planetary arrangements defies easy explanation.
Penn State University researchers uncovered another oddity challenging current understanding of stellar system development. [1] Study of the LHS 3154 system reveals a planet so massive in comparison to its star that generally accepted theories of planet formation cannot explain the existence of the planet, Figure 1. LHS 3154, an “ultracool” star with a “chilly” surface temperature of 2,700 °K (2,430 °C; 4,400 °F), is an M-dwarf, a category that comprises three quarters of the stars in the Milky Way. Most of the light of LHS 3154 is in the infrared band. The M- dwarf star is nine times less massive than the Sun yet it hosts a planet 13 times more massive than Earth.

Figure 1. An artist rendition of the mass comparison between the Earth and Sun and the star LHS 3154, and its companion, LHS 3154b. Credit: Pennsylvania State University.
In current theories, stars form from condensing large clouds of gas and dust into smaller volumes. After the star forms, the left-over gas and dust which is a much smaller fraction of the original cloud, settles into a disk around the new star. From this much smaller mass, planets will condense, completing the star system. In these theories, the star consumes the major proportion of the progenitor clouds.
The Sun, for example, contains an estimated 99.8% of the mass of the Solar System. Only 0.2% is left over for the eight planets, various moons and asteroids.
The mass ratio comparing LHS 3154b to LHS 3154 is 117 times greater than mass ratio comparing the Earth to the Sun. LHS 3154b probably is Neptune-like in composition, completes its orbit in 3.7 Earth days and, the researchers believe, is a very rare world. Typically M-dwarves host small rocky bodies rather than gas giants.
According to current theories, once the star formed, there should not have been enough mass to form a planet as large as LHS 3154b. A young LHS 3154 disk dust-mass and dust-to-gas ratio must be ten times greater than what is typically observed surrounding an M-dwarf star to birth a giant such as LHS 3154b.
“The planet-forming disk around the low-mass star LHS 3154 is not expected to have enough solid mass to make this planet,” Suvrath Mahadevan, the Verne M. Willaman Professor of Astronomy and Astrophysics at Penn State and co-author on the paper said. “But it’s out there, so now we need to reexamine our understanding of how planets and stars form.”
Mahadevan’s team built a novel spectrograph, the Habitable Zone Planet Finder (HPF), with the intention of detecting planets orbiting the coolest of stars. Planets orbiting low temperature stars might have surfaces cool enough to support liquid water and life. In looking for planets with liquid water, the team found, as often happens in research, something new, a massive planet to challenge current theories of stellar system formation.
Another discovery, this time by a Carnegie Institution for Science team, uncovered another challenging world. [2]

Figure 2. Artist’s conception a small red dwarf star, TOI-5205, and its out-sized companion TOI-5205b. Credit: Katherine Cain, the Carnegie Institution for Science.
“The host star, TOI-5205, is just about four times the size of Jupiter, yet it has somehow managed to form a Jupiter-sized planet, which is quite surprising,” observed Shubham Kanodia, who led the team which found TOI-5205b.
When TOI-5205b crosses in front of TOI-5205, the planet blocks about seven percent of the star’s light—a dimming among the largest known exoplanet transit signals.
The rotating disk of gas and dust that surrounds a young star gives birth to its planetary companions. More massive planets require more of the gas and dust left over as the star ignites. Gas planet formation, in the accepted theories, requires about 10 Earth masses of rocky material to produce the massive rocky core of the gas giant. Once the core is formed, it gathers gas from the surrounding clouds, resulting in the mammoth atmosphere of the giant planet.
“TOI-5205b’s existence stretches what we know about the disks in which these planets are born,” Kanodia explained. “In the beginning, if there isn’t enough rocky material in the disk to form the initial core, then one cannot form a gas giant planet. And at the end, if the disk evaporates away before the massive core is formed, then one cannot form a gas giant planet. And yet TOI-5205b formed despite these guardrails. Based on our nominal current understanding of planet formation, TOI-5205b should not exist; it is a ‘forbidden’ planet.”
Not all mysteries are confined to M-dwarfs. A sun-like star, an infant of 14 million years some 360 light years from Earth, hosts a gas giant six times more massive than Jupiter, that orbits the star at a distance twenty times greater than the distance separating Jupiter and the Sun, Figure 3. [3]
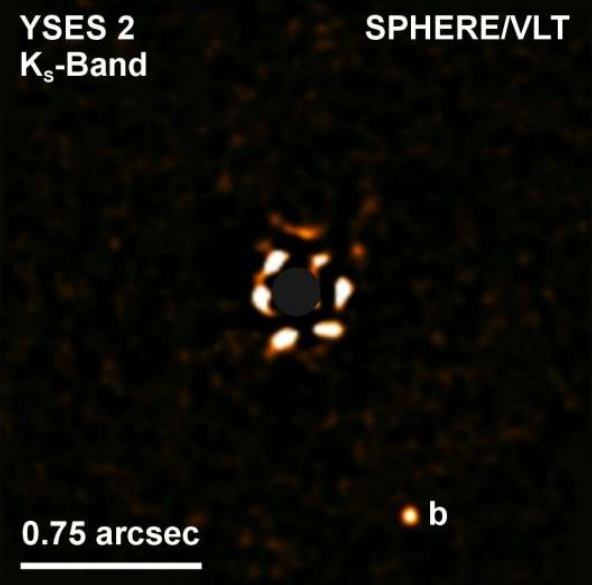
Figure 3. A direct image of the exoplanet YSES 2b (bottom right) and its star (center). The star is blocked by a coronagraph. Credit: ESO/SPHERE/VLT/Bohn et al.
The large distance from YSES 2b to the star does not fit either of the two most well-known models describing large gaseous planet formation. If YSES 2b formed by means of core accretion at such an enormous distance far from the star, the planet should be much lighter than what is observed as a result of scarcity of disk material at that distant location. YSES 2b is too massive to satisfy this theory.
Gravitationally instability, the second theorized method for producing gas giants, postulates very massive protostellar disks that are unstable, splintering into large clumps from which gas giants are directly formed. YSES 2b appears not massive enough to have been formed in this fashion.
In a third possibility, YSES 2b might have formed by core accretion much closer to its host star and migrated outwards. A second planet is needed to pull YSES 2b into the outer regions of the system, but no such planet has been located.
Observations by the current generation of space-borne telescopes have upset the theories of planet formation. Hot Jupiters, worlds orbiting pulsars, odd arrangements of worlds, super Earths, and wandering worlds flung close to a star then flying back have complicated the ideas of Laplace, See, Chamberlin and Moulton. Further study by the James Webb Space Telescope and its successors will only enliven the debate surrounding the origin of the planets.
References
[1] Guðmundur Stefánsson, Suvrath Mahadevan, Yamila Miguel, et al, “A Neptune-mass exoplanet in close orbit around a very low-mass star challenges formation models,” Science, 30 Nov 2023, Vol. 382, Issue 6674, pp. 1031-1035, DOI: 10.1126/science.abo0233.
[2] Shubham Kanodia et al, “TOI-5205b: A Short-period Jovian Planet Transiting a Mid-M Dwarf,” The Astronomical Journal (2023). DOI: 10.3847/1538-3881/acabce
[3] Alexander J. Bohn et al. “Discovery of a directly imaged planet to the young solar analog YSES 2.” Accepted for publication in Astronomy & Astrophysics, www.aanda.org/10.1051/0004-6361/202140508

Interstellar Precursor? The Statite Solution

What an interesting object Methone is. Discovered by the Cassini imaging team in 2004 along with the nearby Pallene, this moon of Saturn is a scant 1.6 kilometers in radius, orbiting between Mimas and Enceladus. In fact, Methone, Pallene and another moon called Anthe all orbit at similar distances from Saturn and are dynamically jostled by Mimas. What stands out about Methone is first of all its shape and, perhaps even more strikingly, the smoothness of its surface. We’d like to know what produces this kind of object and would also like to retrieve imagery of both Pallene and Anthe. If something this strange has equally odd companions, is there something about its relationship with both nearby moons and Saturn’s rings that can produce this kind of surface?
Image: It’s difficult not to think of an egg when looking at Saturn’s moon Methone, seen here during a Cassini flyby of the small moon. The relatively smooth surface adds to the effect created by the oblong shape. NASA/JPL-Caltech/Space Science Institute.
Our path to interstellar missions will see us ramp up the velocities of our probes to objects in our own system, made more accessible by shorter mission times, sail technologies and miniaturization. There is no shortage of targets between high-interest moons like Europa, Titan and Enceladus and Kuiper Belt Objects like Arrokoth. For that matter, the interstellar interloper ‘Oumuamua may yet be within range of faster missions (and in fact we’ll be examining ‘Oumuamua prospects in at least one upcoming article). But the point is that intermediate steps to interstellar will enhance exploration of objects we’ve already visited and take us to numerous others.
One way to proceed is discussed by Greg Matloff and Les Johnson in a recent paper for the Journal of the British Interplanetary Society that grew out of a presentation at the 6th International Space Sailing Symposium this summer. Here the idea is to adjust the parameters of a solar sail so that a balance is achieved between the gravitational force of the Sun and the solar photon radiation impinging upon it. The parameters are clear enough: We need a sail of a specific thickness (areal density), and tightly constrained figures for its reflectance and absorbance. We want to cancel out the gravitational acceleration imposed by the Sun through the propulsive effects of solar photons, allowing us to effectively ‘hover’ in place.
Hovering isn’t traveling, but bear with me. We’ve looked at this kind of sail configuration before and discussed its development in the hands of Robert Forward. It was Forward who dubbed the configuration a ‘statite,’ implying that when the force on the sail from solar radiation exactly balances the gravitational force acting upon it, the spacecraft is effectively in what the paper calls a ‘force-free environment.’
This gets interesting in terms of fast probes because while the statite is normally considered to remain stationary (and it will do so when the sail is stationary relative to the Sun during sail deployment), something else happens when the craft is orbiting the Sun when the sail is deployed. The sail now moves in a straight line at its orbital velocity at the time of deployment. The authors style this ‘rectilinear sun-diving.’ As Matloff noted in an email the other day:
“To do this operationally, it is necessary to maintain the sail normal to the Sun – broadside facing the Sun – during the acceleration process. The sail moves off at its velocity relative to the Sun at sail deployment because radiation pressure force on the sail balances solar gravitational attraction. This is a consequence of Newton’s First Law.”
Using this method we can fling the sail and payload outward. What is known as the sail’s lightness factor is the ratio of solar radiation forces divided by the solar gravitational force, and in the case of the rectilinear trajectory described above, the lightness factor is 1. So consider a sail being deployed from a circular orbit of the Sun at 1 AU. The statite, free of other forces, now moves out on a rectilinear trajectory at 30 kilometers per second, which is the Earth’s orbital velocity. The number is noteworthy because it practically doubles the interstellar velocity of Voyager 1. Matloff and Johnson point out that at this velocity, the Sun’s gravitational focus at 550 AU is reachable in 87 years.
Moving at the same pace gets us to Saturn (and the interesting Methone) in 1.5 years. I’m going to run through the other two scenarios the scientists consider to show the range of possibilities. Assume an orbit that is not circular but rather one having a perihelion of 0.7 AU and aphelion at 1 AU. Deploying the sail at perihelion allows the spacecraft to reach 38 kilometers per second, getting to the inner gravitational focus in about 66 years. Finally, with an aphelion at 1 AU and perihelion at 0.3, our craft achieves a velocity after sail deployment of 66 km/sec, reaching the focus in 38 years.
As regards to ‘Oumuamua, the third scenario, with sail deployment at perihelion some 0.3 AU out from the Sun, achieves enough interstellar cruise velocity to catch the object roughly around 2045, when it will be some 220 AU from the Sun. To these times, of course, must be added the time needed to move the sail from aphelion to the sail deployment point at perihelion, but the numbers are still quite satisfactory.
This is especially true given that we are talking about relatively near-term technologies that are under active development. Matloff and Johnson calculate using an areal mass thickness of 1.46 X 10-3kg/m2 for the proposed missions. They show current state of the art solar sail film as 1.54 X 10-3kg/m2 (this does not include deployment mechanisms, structure, etc). The point is clear, however: Achieving 30 km/sec or more offers us fast passage to targets within the outer Solar System as we analyze options for missions beyond it, using technologies that are not far removed from present capability.
The authors note that we can’t assume a constant value for solar radiation; the solar constant actually varies by about 0.1% in response to the Sun’s activity cycle. Hence the need to explore options like adjusting the curvature of the sail or using reflective vanes for fine-tuning. Controlling the sail will obviously be critical. The paper continues:
Control of the sail depends upon the ability of the system to dynamically adjust the center of mass (CM) versus the center of (photon) pressure (CP). Any misalignment of the CM versus the CP will induce torques in the sail system that have to be actively managed lest the offset result in an eventual loss of control. The sail will encounter micrometeorites and interplanetary dust during flight that will create small holes in the fabric, changing its reflectivity asymmetrically and inducing unwanted torques. Depending upon how the sail is packaged and deployed, there may also be fold lines, wrinkles, and small tears that occur with similar end results.
Hence the need for a momentum management system, which could involve possibilities like reflective control devices for roll or diffractive sail materials that manipulate the exit direction of incoming photons as needed to counter these effects. The authors point out that the solar sail propulsion systems for this kind of mission are at TRL-6 despite recent failures such as the loss of the Near-Earth Asteroid Scout Cubesat mission, which carried an 86 square meter solar sail that was lost after launch in late November 2022. With solar sails under active development, however, the prospect for exploring rectilinear sundiver missions in the near term seems quite plausible.
The paper is Matloff & Johnson, “Breakthrough Sun Diving: The Rectilinear Option,” Journal of the British Interplanetary Society Vol. 76 (2023), 283-287.

SETI: Musings on the Barrow Scale
John Barrow has been on my mind these past few days, for reasons that will become apparent in a moment. In my eulogy for Barrow (1952-2020), I quoted from his book The Left Hand of Creation (Oxford, 1983). I want to revisit that passage for its clarity, something that always inspired me about this brilliant physicist. For it seemed he could render the complex not only accessible but encouragingly pliable, as if scientific exploration always unlocked doors of possibility we could use to our advantage. His was a bright vision. The notion that animated him was that there was something in the sheer process of research that held its own value. Thus:
Could there be any shortcuts to the answers to the cosmological questions? There are some who foolishly desire contact with advanced extraterrestrials in order that we might painlessly discover the secrets of the universe secondhand and prematurely extend our understanding. Such a civilization would surely resemble a child who receives as a gift a collection of completed crossword puzzles. The human search for the structure of the universe is more important than finding it because it motivates the creative power of the human imagination.
You can see that for Barrow, the question of values was not separated from scientific results, and in a sense transcended the data we actually gathered. He goes on:
About 50 years ago a group of eminent cosmologists were asked what single question they would ask of an infallible oracle who could answer them with only yes or no. When his opportunity came, Georges Lemaître made the wisest choice. He said, “I would ask the Oracle not to answer in order that a subsequent generation would not be deprived of the pleasure of searching for and finding the solution.”

Image: Cosmologist, mathematician and physicist John D. Barrow, whose books have been a personal inspiration for many years. Credit: Tom Powell.
Leave it to Lemaître (and Barrow to quote him) as we reach beyond the immediately practical to unlock what it is about human experience that compels us to push into new terrain, whether it be physical exploration or flights of the imagination as we pursue a new hypothesis about nature. Barrow comes to mind because we’ve just been talking about the scales by which a civilization can be measured. Some of these are well established, as for example the Kardashev scale, with its familiar Types I, II and III keyed to the scale of a civilization’s energy use. In Clarke’s The Fountains of Paradise we find an alien scale based on the use of tools. It’s possible to imagine other scales, and Barrow’s own contribution takes us into the nano-realm.
As best I can determine, Barrow first floated the scale in his 1998 book Impossibility: The limits of science and the science of limits (Oxford University Press). Inverting Kardashev, Barrow was interested in a civilization’s ability to control smaller and smaller things, relying on the observed fact that as we have explored such micro-realms, our technologies have proliferated. Nanotechnology and biotechnology are drawn out of our ability to manipulate matter at small scales, and in fact the development of nanotech is one marker for a Barrow scale IV culture.
Barrow I: The ability to manipulate objects at the same scale as the person or being involved. In other words, simple activities involving basic tools.
Barrow II: The control of genetic information.
Barrow III: The ability to control molecules.
Barrow IV: The ability to control individual atoms.
Barrow V: The manipulation of atomic nuclei..
Barrow VI: Control of elementary particles.
Barrow Omega (Ω): The ability to control fundamental elements of spacetime.
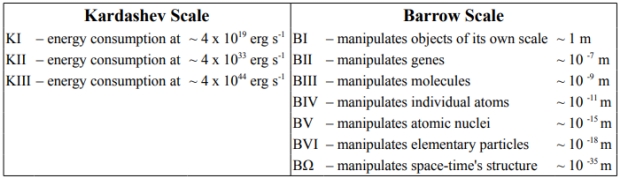
Table: Energetic and inward civilization development. Kardashev’s (1964) types refer to energy consumption; Barrow’s (1998, 133) types refer to a civilization’s ability to manipulate smaller and smaller entities. Credit: Clément Vidal.
I’ve drawn the above table from a paper by French philosopher and SETI scientist Clément Vidal, who is one of the few who have explored this realm (citation below). Here we get both Kardashev and Barrow at once, a convenience, and central to Vidal’s argument that black holes are going to draw advanced civilizations to extract their energies and explore what he calls “the computational density of matter.” On this score, it’s interesting to note that Freeman Dyson proposed in 1979 that a civilization exploiting time dilation effects near black holes could survive effectively forever (a later revision had to take into account the accelerating expansion of the universe).
What all this means for SETI is intriguing – almost punchy – and I’ll send you to Vidal’s superb The Beginning and the End: The Meaning of Life in a Cosmological Perspective (Springer, 2014) for a deep dive into the concepts involved. But consider this for a starter: Dysonian SETI assumes civilizations far more advanced than our own, the reasoning being that their works should be apparent even at astronomical scales. Thus searching our astronomical data as far back as we can could conceivably flag an anomaly that merits investigation as a possible civilizational marker.
What Clément Vidal has been investigating is where such markers would turn up, and for this he deploys the scales of both Kardashev and Barrow. I think the easiest assumption is that we would find an alien civilization at its home world, but of course this needn’t be the case. Vidal speaks of ‘attractors’ as those sources of energy that an advanced civilization would increasingly exploit. Take a culture a billion years older than our own and ponder energy needs that might require it to exploit things like the energies of close binary neutron stars or black holes themselves. Such a civilization would be far flung, with operations well beyond its local group of stars.
Now ponder Barrow Type Ω. This ‘omega’ culture is free of the constraints of spacetime, having achieved the ability to manipulate both. It’s anyone’s guess whether such a civilization would be noted by achievements on a truly celestial scale, or whether its works would actually be embedded in the nature of space and time themselves, so that to us they appear the simple functioning of nature. In this mode of thinking, the more advanced a civilization becomes as it moves up the Barrow scale, the more it begins to effectively disappear. Barrow thus channels Richard Feynman and anticipates Lee Smolin’s notions about cosmological evolution, a kind of self-selection for universes.
I’m going to swipe the chart below from Vidal’s 2010 paper on black hole attractors, showing the entertaining fact that as he puts it, “from the relative human point of view, there is more to explore in small scales than in large scales.”
Table: That humans are not in the center of the universe is also true in terms of scales. This implies that there is more to explore in small scales than in large scales. Richard Feynman (1960) popularized this insight when he said “there is plenty of room at the bottom”. Figure adapted from (Auffray and Nottale 2008, 86). Credit: Clément Vidal.
Futurist John Smart has dug into what he calls STEM Compression, with STEM in this case meaning Space/Time/Energy/Matter, and the compression being the idea that in terms of density and efficiency, we can as Vidal puts it “do more with less.” For going deeper into the Barrow scale, we see that as things get smaller, we are not hampered by the speed of light problem. In fact, our endgame barrier is at the Planck scale. A Kardashev II civilization extracting energy from a rotating black hole using technologies far up the Barrow scale may well be indistinguishable from an X-ray binary of the sort that has been cataloged in the astronomical literature.
Such speculations are on the far edge of SETI (and again, I refer you to Vidal’s book), but it’s also true that whether or not extraterrestrial civilizations exist, our own ability to chart futures for an expanding civilization may well come in handy if we can somehow punch through whatever ‘great filter’ may be out there and become a species that survives on the scale of deep time. There is no knowing whether this is even possible, and it may be that the galaxy is filled with the ruins of those who have gone before us.
It is also true, of course, that no one may have gone before us. Maybe N really does equal 1. But I return to Barrow: “The human search for the structure of the universe is more important than finding it because it motivates the creative power of the human imagination.” And the human imagination is currency of the realm in matters like these.
The Vidal paper is “Black Holes: Attractors for Intelligence?” presented at the Kavli Royal Society International Centre, “Towards a scientific and societal agenda on extra-terrestrial life”, 4-5 Oct 2010 (abstract). The Dyson paper is “Time Without End: Physics and Biology in an Open Universe,” Review of Modern Physics 51: 447-460 (abstract). My eulogy for Barrow is On John Barrow. John Smart contributed a fascinating essay on cosmic evolution in these pages in The Goodness of the Universe.

Talking to Starglider
When we’ve discussed interstellar ‘interlopers’ like ‘Oumuamua and 2I/Borisov, the science fiction-minded among us have now and then noted Arthur Clarke’s Rendezvous with Rama (Gollancz, 1973). Although we’ve yet to figure out definitively what ‘Oumuamua is (2/I Borisov is definitely a comet), the Clarke reference is an imaginative nod to the possibility that one day an alien craft might enter our Solar System during a gravitational assist maneuver and be flung outward on whatever its mission was (in Rama’s case, out in the direction of the Large Magellanic Cloud).

Since we’ll never see ‘Oumuamua again, we wait with great anticipation the work of the Legacy Survey of Space and Time (LSST), which will be run via the Vera Rubin Telescope (first light in 2025). Estimates vary widely but the consensus seems to be that with a telescope capable of imaging the entire visible sky in the southern hemisphere every few nights, the LSST should produce more than a few interstellar objects, perhaps ten or more, every year. We probably won’t find a Rama, but who knows?
Meanwhile, I’m reminded of another Clarke novel that rarely gets the attention in this regard that Rendezvous with Rama does. This is 1979’s The Fountains of Paradise (BCA/Gollancz). Although known primarily for its exploration of space elevators (and its reality-distorting geography), the novel includes as a separate theme another entry into the Solar System, this time by a craft that, unlike Rama, is willing to take notice of us. Starglider is its name, and it represents a civilization that is cataloging planetary systems through probes scattered across a host of nearby stars.
Starglider has a 500 kilometer antenna to communicate with its home star (humans name this Starholme), and in the words of a report on its activities within the novel, it more or less ‘charges its batteries’ each time it makes a close stellar pass. Having explored the Alpha Centauri trio, its next destination after the Sun is Tau Ceti. The game plan is that each stellar encounter will gather data and open communications with any civilization found there as a precursor to long-term radio contact and, presumably, entry into some kind of interstellar information network.
This is rather fascinating. For Starglider is smart enough to have studied human languages and is able to converse, after a fashion. From the novel:
It was obvious from its first messages that Starglider understood the meaning of several thousand basic English and Chinese words, which it had deduced from an analysis of television, radio, and especially broadcast video-text services. But what it had picked up during its approach was a very unrepresentative sample from the whole spectrum of human culture; it contained little advanced science, still less advanced mathematics, and only a random selection of literature, music, and the visual arts.
Like any self-taught genius,therefore, Starglider had huge gaps in its education. On the principle that it was better to give too much than too little, as soon as contact was established, Starglider was presented with the Oxford English Dictionary, the Great Chinese Dictionary (Mandarin edition), and the Encyclopedia Terrae. Their digital transmission required little more than fifty minutes, and it was notable that immediately thereafter Starglider was silent for almost four hours — its longest period off the air. When it resumed contact, its vocabulary was immensely enlarged, and more than ninety-nine percent of the time it could pass the Turing test with ease — that is, there was no way of telling from the messages received that Starglider was a machine, and not a highly intelligent human.
Clarke slyly notes the cultural differences between species as opposed to the commonality of, say, mathematics, saying that Starglider had little comprehension of lines like this from Keats:
Charm’d casements, opening on the foam
Of perilous seas, in faery lands forlorn…
And it drew a blank on Shakespeare as well:
Shall I compare thee to a summer’s day?
Thou art more lovely and more temperate…
Well, these are aliens, after all. We have enough trouble with cross-cultural references here on Earth. Humans broadcast thousands of hours of music and video drama to Starglider to help it out, but here, of course, we run into the messaging problem. Just how much do we want to reveal of ourselves to a culture about which we have all too little information other than that it is markedly more advanced than our own? You’ll find that aspect of the METI debate explored as a core part of the Starglider subplot.
Some have panned Starglider’s appearance in the novel because it seems intrusive to the plot (although I suppose I could argue that autonomous probes cataloging stellar systems almost have to be intrusive to get their job done). But in the midst of the Starglider passages, we learn that the chatty aliens, now freely talking to humans via radio, catalog the civilizations they find on a scale based on their technological accomplishments. Is this Clarke channeling Nikolai Kardashev?
Whatever the case, Clarke as always takes the long view, and the long view by its very nature always pushes out into mystery. Consider the scale used by Starglider:
I. Stone Tools
II. Metals, fire
III. Writing, handicrafts, ships
IV. Steam power, basic science
V. Atomic energy, space travel
VI. “…the ability to convert matter completely into energy, and to transmute all elements on an industrial scale.”
On this scale of one through six we can place our species at level 5, as Starglider sees us. But are there further levels? Clarke is wise to imply their existence without exploring it any further, as this lets the reader’s imagination do the job. He’s expert at this:
“And is there a Category Seven?” Starglider was immediately asked. The reply was a brief “Affirmative.” When pressed for details, the probe explained: “I am not allowed to describe the technology of a higher-grade culture to a lower one.” There the matter remained, right up to the moment of the final message, despite all the leading questions designed by the most ingenious legal brains of Earth.
When the University of Chicago’s Department of Philosophy transmits the whole of Thomas Aquinas’ Summa Theologica to Starglider, all hell breaks loose. I turn you to the novel for more.

Image; Hubble took this image on Oct. 12, 2019, when comet 2I/Borisov was about 418 million kilometers from Earth. The image shows dust concentrated around the nucleus, but the nucleus itself was too small to be seen by Hubble. We are on the cusp of a windfall of ‘interstellar interloper’ data as the LSST comes online within a few years. Will we ever find a Rama, or a Starglider, amidst our observations? Credit: NASA, ESA and D. Jewitt (UCLA).
As I mentioned, some critics fault The Fountains of Paradise for Starglider’s very presence, noting that there are essentially two plots at work here. In fact there are in fact three plots taking place on different timescales here, one of them dating back several thousand years, and recall that the voyage of Starglider itself spans millennia, the mission having began some 60,000 years before the events of the main part of the novel – construction of the space elevator – take place. This kind of chronological juggling, allows Clarke to inspire deeper reflection on humanity’s place in the universe and I find it enormously effective.
Wonders fairly pop out of Clarke’s early novels and much of his later work. On that score, I likewise refuse to fault him severely because he cannot achieve complex characterization. A case can be made (James Gunn makes it strongly) that science fiction of Clarke’s ilk needs to put the wonder first. Rich, strange and complicated characters confronting rich, strange and wondrous events may lead to one richness too many. For we, the readers, to absorb the mystery, we need to see how a relatively straightforward character reacts. It’s that contrast that Clarke aims to mine.
That’s only one way of doing science fiction, but much science fiction of the 1950s, which I consider the genre’s true golden age (with a nod to the late 1930s, as one must) often operated with precisely this conceit. And that’s okay, because when writers of greater literary style began to emerge – writers like Alfred Bester, say, with his staggering The Stars My Destination (1956) we were able to see complex characters confronting the deeply strange in ways that simply added depth to the experience. Look at Robert Silverberg in the 1960s as an exemplar of an almost magical insight into what makes the individual human tick. Once you’ve begun on that journey, the field is altered forever, but that doesn’t negate its rich past.
In fact, none of this subsequent growth nullifies Clarke’s accomplishment in the realm of big ideas. Consider him a writer of a kind of SF that flourished and fed a mighty stream into what has now become a river of wildly untamed ideas and insights. And sometimes only Clarke will do. Thus when i read, for the umpteenth time, The City and the Stars, I’m again dazzled by the very title, and the first few pages take me back into a realm where there are suns not quite our own casting a numinous glow over landscapes we learn to navigate through characters who learn with us. Like Stapledon’s, like Asimov’s, Clarke’s is a voice we’ll celebrate deep into the future.


